Advertisements
Advertisements
प्रश्न
Prove that the tangent drawn at the mid-point of an arc of a circle is parallel to the chord joining the end points of the arc.
उत्तर
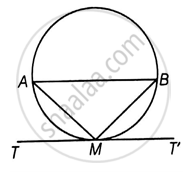
Let us draw a circle in which AMB is an arc and M is the mid-point of the arc AMB.
Joined AM and MB.
Also TT' is a tangent at point M on the circle.
To Prove: AB || TT'
Proof: As M is the mid point of Arc AMB
Arc AM = Arc MB
AM = MB ...[As equal chords cuts equal arcs]
∠ABM = ∠BAM ...[Angles opposite to equal sides are equal] [1]
Now, ∠BMT' = ∠BAM ...[Angle between tangent and the chord equals angle made by the chord in alternate segment] [2]
From [1] and [2]
∠ABM = ∠BMT'
So, AB || TT' ...[Two lines are parallel if the interior alternate angles are equal]
Hence Proved!
APPEARS IN
संबंधित प्रश्न
The common point of a tangent to a circle and the circle is called ______.
In triangle PQR, PQ = 24 cm, QR = –7 cm and ∠PQR = 90°. Find the radius of the inscribed circle.

In Figure 5, a triangle PQR is drawn to circumscribe a circle of radius 6 cm such that the segments QT and TR into which QR is divided by the point of contact T, are of lengths 12 cm and 9 cm respectively. If the area of ΔPQR = 189 cm2, then find the lengths of sides PQ and PR.
In the given figure AC is a tangent to the circle with centre O.
If ∠ADB = 55° , find x and y. Give reasons for your answers.

PA and PB are tangents from P to the circle with centre O. At M, a tangent is drawn cutting PA at K and PB at N. Prove that KN = AK + BN.
In the given figure, O is the centre of the circle. Tangents at A and B meet at C. If angle ACO = 30°, find: angle APB

In the figure, if PA and PB are tangents to the circle with centre O such that ∠APB = 50°, then ∠OAB is equal to ______.

In Question 5 above, if radii of the two circles are equal, prove that AB = CD.
ΔABC circumscribes a circle of radius r such that ∠B = 90°. If AB = 3 cm and BC = 4 cm, then find the value of r.
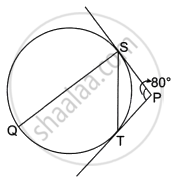
In the given diagram, PS and PT are the tangents to the circle. SQ || PT and ∠SPT = 80°. The value of ∠QST is ______.