Advertisements
Advertisements
प्रश्न
In Question 5 above, if radii of the two circles are equal, prove that AB = CD.
उत्तर
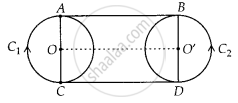
Join OO’
Since, OA = O’B ...[Given]
Also, ∠OAB = ∠O’BA = 90° ...[Tangent at any point of a circle is perpendicular to the radius at the point of contact]
Since, perpendicular distance between two straight lines at two different points is same.
⇒ AB is parallel to OO’
Similarly, CD is parallel to OO’
⇒ AB ॥ CD
Also, ∠OAB = ∠OCD = ∠O’BA = ∠O’DC = 90°
⇒ ABCD is a rectangle.
Hence, AB = CD.
APPEARS IN
संबंधित प्रश्न
A line intersecting a circle in two points is called a ______.
A chord PQ of a circle is parallel to the tangent drawn at a point R of the circle. Prove that R bisects the arc PRQ.
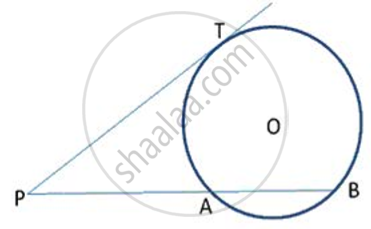
In following fig., PT is a tangent to the circle at T and PAB is a secant to the same circle. If PA = 4cm and AB = Scm, find PT.
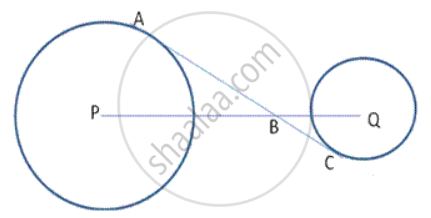
In the following fig. , AC is a transversal common tangent to tvvo circles with centres P and Q and of radii 6cm and 3cm respectively. Given that AB = 8cm, calculate PQ.
Construct a tangent to a circle with centre O and radius 3.5 cm at a point P on it.
In figure, O is the centre of a circle of radius 5 cm, T is a point such that OT = 13 cm and OT intersects the circle at E. If AB is the tangent to the circle at E, find the length of AB.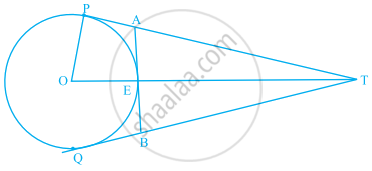
Construct a pair of tangents to a circle of radius 4 cm, which are inclined to each other at an angle of 60°.
In the given figure, PQ is a tangent to the circle with centre O. If ∠OPQ = x, ∠POQ = y, then x + y is ______.

In the given figure, PT is a tangent at T to the circle with centre O. If ∠TPO = 25°, then x is equal to ______.

In the given figure O, is the centre of the circle. CE is a tangent to the circle at A. If ∠ABD = 26° find:
- ∠BDA
- ∠BAD
- ∠CAD
- ∠ODB

