Advertisements
Advertisements
प्रश्न
Construct a pair of tangents to a circle of radius 4 cm, which are inclined to each other at an angle of 60°.
उत्तर

Steps of construction:
- Draw a circle with radius 4 cm with the centre as O.
- Take a point A on the circumference of the circle and join OA.
- Draw a radius OB, making an angle of 120° (180° – 60°) with OA.
- Draw a perpendicular to OB at point B and perpendicular to OA at point A. Let both perpendiculars intersect at point P.
PA and PB are the required tangents at an angle of 60°.
APPEARS IN
संबंधित प्रश्न
How many tangents can a circle have?
In Figure 3, a right triangle ABC, circumscribes a circle of radius r. If AB and BC are of lengths of 8 cm and 6 cm respectively, find the value of r.

Two chords AB and CD of lengths 6cm and 12cm are drawn parallel inside the circle. If the distance between the chords of the circle is 3cm, find the radius of the circle.
Find the length of the tangent from a point which is at a distance of 5cm from the centre of the circle of radius 3cm.
In the given figure, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find the length of tangent PT.

In figure, if ∠AOB = 125°, then ∠COD is equal to ______.

In figure, O is the centre of a circle of radius 5 cm, T is a point such that OT = 13 cm and OT intersects the circle at E. If AB is the tangent to the circle at E, find the length of AB.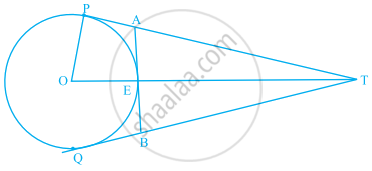
The distance between two tangents parallel to each other of a circle is 13 cm. Find the radius of the circle.
The length of tangent drawn to a circle of radius 9 cm from a point 41 cm from the centre is ______.
If O is centre of a circle and Chord PQ makes an angle 50° with the tangent PR at the point of contact P, then the angle subtended by the chord at the centre is ______.