Advertisements
Advertisements
प्रश्न
In the given figure O, is the centre of the circle. CE is a tangent to the circle at A. If ∠ABD = 26° find:
- ∠BDA
- ∠BAD
- ∠CAD
- ∠ODB

उत्तर
Given ∠ABD = 26°
a. Angle in semi-circle is a right angle
∴ ∠BDA = 90°

b. Sum of all angles in a triangle = 180°
∴ ∠BAD + ∠BDA + ∠ABD = 180°
∠BAD + 90° + 26° = 180°
∠BAD = 180° – 116°
= 64°
c. Angles in alternate segments are equal
∴ ∠CAD = ∠ABD
= 26°
d. In ΔODB,
OB = OD ...(Radii of same circle)
∴ ∠ODB = ∠OBD
= ∠ABD
= 26°
APPEARS IN
संबंधित प्रश्न
The given figure shows a circle with centre O and BCD is tangent to it at C. Show that : ∠ACD + ∠BAC = 90°.

How many common tangents can be drawn to two circles, touching each
other externally?
Draw Δ ABC such that, AB = 8 cm, BC = 6 cm and ∠ B = 90°. Draw seg BD
perpendicular to hypotenuse AC. Draw a circle passing through points
B, D, A. Show that line CB is a tangent of the circle.
AB is a diameter and AC is a chord of a circle with centre O such that ∠BAC = 30°. The tangent at C intersects extended AB at a point D. Prove that BC = BD.
In following fig., PT is a tangent to the circle at T and PAB is a secant to the same circle. If PB = 9cm and AB = Scm, find PT.
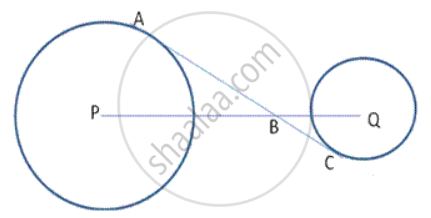
In the following fig. , AC is a transversal common tangent to tvvo circles with centres P and Q and of radii 6cm and 3cm respectively. Given that AB = 8cm, calculate PQ.
In the alongside, figure, O is the centre of the circumcircle of triangle XYZ. Tangents at X and Y intersect at T. Given ∠XTY = 80° and ∠XOZ = 140°. Calculate the value of ∠ZXY.

Tangents from an external point to a circle are ______
In the given figure, PT is a tangent at T to the circle with centre O. If ∠TPO = 25°, then x is equal to ______.

In the adjoining figure, PT is a tangent at T to the circle with centre O. If ∠TPO = 30°, find the value of x.

