Question

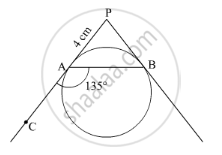
In the given figure, PA and PB are tangents to a circle from an external point P such that PA = 4 cm and ∠BAC = 135°. Find the length of chord AB ?

Answer 1

It is given that PA and PB are tangents drawn from an external point P to the circle.

∴ PA = PB = 4 cm     (Lengths of tangents drawn from an external point to a circle are equal)

Also, \[\angle BAC = 135^o\]

Now,

\[\angle BAC + \angle PAB = 180^o\]           (Linear pair of angles)

\[\therefore 135^o + \angle PAB = 180^o\]
\[ \Rightarrow \angle PAB = 180^o - 135^o = 45^0\]

In ∆PAB,

PA = PB

∴ \[\angle PBA = \angle PAB = 45^o\]         (In a triangle, equal sides have equal angles opposite to them)

Also,

\[\angle PBA + \angle PAB + \angle APB = 180^o\]      (Angle sum property)

\[\Rightarrow 45^O + 45^o + \angle APB = 180^o\]
\[ \Rightarrow \angle APB = 180^o - 90^o = 90^o\]

So, ∆PAB is a right triangle right angled at P.

Using Pythagoras theorem, we have

\[{AB}^2 = {PA}^2 + {PB}^2 \]
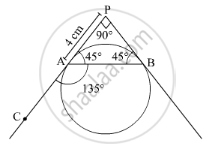
\[ \Rightarrow AB = \sqrt{\left( 4 \right)^2 + \left( 4 \right)^2} = \sqrt{32} = 4\sqrt{2} cm\]

Thus, the length of the chord AB is

\[4\sqrt{2} cm .\]