Advertisements
Advertisements
प्रश्न
In a series LCR circuit, obtain the condition under which watt-less current flows in the circuit ?
उत्तर
Average power dissipated through a series LCR circuit is given by \[P_{av} = E_v I_v \cos\left( \phi \right)\]
where, Ev = rms value of alternating voltage
Iv = rms value of alternating current
Φ = phase difference between current and voltage
For wattless current, the power dissipated through the circuit should be zero.
\[i . e . , \cos\left( \phi \right) = 0\]
\[ \Rightarrow \phi = \frac{\pi}{2}\]
Hence, the condition for wattless current is that the phase difference between the current and voltage should be π/2 and the circuit is purely inductive or purely capacitive.
APPEARS IN
संबंधित प्रश्न
In a series LCR circuit, obtain the condition under which the impedance of the circuit is minimum ?
An LR circuit contains an inductor of 500 mH, a resistor of 25.0 Ω and an emf of 5.00 V in series. Find the potential difference across the resistor at t = (a) 20.0 ms, (b) 100 ms and (c) 1.00 s.
An inductor-coil of resistance 10 Ω and inductance 120 mH is connected across a battery of emf 6 V and internal resistance 2 Ω. Find the charge which flows through the inductor in (a) 10 ms, (b) 20 ms and (c) 100 ms after the connections are made.
An inductor-coil of inductance 17 mH is constructed from a copper wire of length 100 m and cross-sectional area 1 mm2. Calculate the time constant of the circuit if this inductor is joined across an ideal battery. The resistivity of copper = 1.7 × 10−8 Ω-m.
To reduce the resonant frequency in an LCR series circuit with a generator
Consider the LCR circuit shown in figure. Find the net current i and the phase of i. Show that i = v/Z`. Find the impedance Z for this circuit.
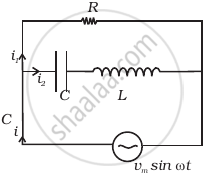
For an LCR circuit driven at frequency ω, the equation reads
`L (di)/(dt) + Ri + q/C = v_i = v_m` sin ωt
- Multiply the equation by i and simplify where possible.
- Interpret each term physically.
- Cast the equation in the form of a conservation of energy statement.
- Integrate the equation over one cycle to find that the phase difference between v and i must be acute.
A series LCR circuit driven by 300 V at a frequency of 50 Hz contains a resistance R = 3 kΩ, an inductor of inductive reactance XL = 250 πΩ, and an unknown capacitor. The value of capacitance to maximize the average power should be ______.
Select the most appropriate option with regard to resonance in a series LCR circuit.
