Advertisements
Advertisements
प्रश्न
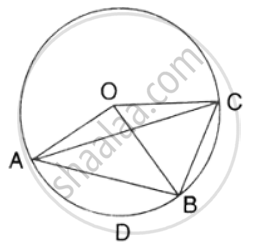
In the figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC. If angle AOB = 108°, find: ∠ADB.
उत्तर

Again, Arc AB subtends ∠AOB at the centre and
∠ACB at the remaining part of the circle.
∠ ACB = `1/2` ∠AOB
=` 1/2 xx 108°`
= 54
In cyclic quadrilateral ADBC
∠ADB + ∠ACB = 180° ...[sum of opposite angles]
⇒ ∠ADB + 54° = 180°
⇒ ∠ADB = 180° − 54°
⇒ ∠ADB = 126°
APPEARS IN
संबंधित प्रश्न
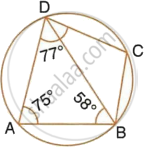
In the figure, given below, ABCD is a cyclic quadrilateral in which ∠BAD = 75°; ∠ABD = 58° and ∠ADC = 77°. Find:
- ∠BDC,
- ∠BCD,
- ∠BCA.
In cyclic quadrilateral ABCD, ∠DAC = 27°; ∠DBA = 50° and ∠ADB = 33°.
Calculate:
- ∠DBC,
- ∠DCB,
- ∠CAB.

In a square ABCD, its diagonals AC and BD intersect each other at point O. The bisector of angle DAO meets BD at point M and the bisector of angle ABD meets AC at N and AM at L. Show that:
- ∠ONL + ∠OML = 180°
- ∠BAM + ∠BMA
- ALOB is a cyclic quadrilateral.
In the given figure, AB is parallel to DC, ∠BCE = 80° and ∠BAC = 25°.

Find:
- ∠CAD
- ∠CBD
- ∠ADC
In a cyclic quadrialteral ABCD , if m ∠ A = 3 (m ∠C). Find m ∠ A.
ABCD is a cyclic quadrilateral, AB and DC are produced to meet in E. Prove that ΔEBC ≅ ΔEDA.
In following figure.,ABCD is a cyclic quadrilateral . If ∠ BCD = 100° and ∠ ABD = 70° , find ∠ ADB.
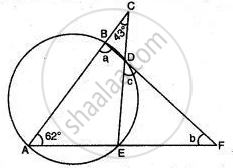
In the following figure, O is the centre of the circle. Find the values of a, b and c.

In the figure, ∠DBC = 58°. BD is a diameter of the circle. Calculate : ∠BEC

In the given figure, if ∠ ACE = 43° and ∠CAF = 62°. Find the value of a, b, and c.