Advertisements
Advertisements
प्रश्न
In following figure.,ABCD is a cyclic quadrilateral . If ∠ BCD = 100° and ∠ ABD = 70° , find ∠ ADB.
उत्तर
In cyclic quadrilateral ABCD,
∠ BCD + ∠ DAB = 180° (Opposite angles of a cyclic quadrilateral)
100 + ∠ DAB = 180
∠ DAB = 80°
In Δ DAB ,
∠ DAB + ∠ ABD + ∠ BDA = 180°
80 + 70° + ∠ BDA = 180°
∠ BDA = 30°
APPEARS IN
संबंधित प्रश्न
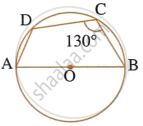
In the given figure, AB is the diameter of a circle with centre O. ∠BCD = 130o. Find:
1) ∠DAB
2) ∠DBA

In the figure, ABCD is a cyclic quadrilateral with BC = CD. TC is tangent to the circle at point C and DC is produced to point G. If ∠BCG = 108° and O is the centre of the circle, find :
- angle BCT
- angle DOC

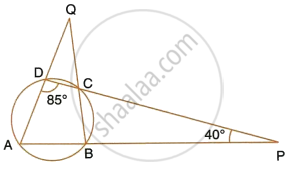
Use the given figure to find:
- ∠BAD,
- ∠DQB.
ABCD is a cyclic quadrilateral. Sides AB and DC produced meet at point E; whereas sides BC and AD produced meet at point F. If ∠DCF : ∠F : ∠E = 3 : 5 : 4, find the angles of the cyclic quadrilateral ABCD.
In a circle with centre O , chords AB and CD intersets inside the circle at E . Prove that ∠ AOC = ∠ BOD = 2 ∠ AEC.
Prove that the angles bisectors of the angles formed by producing opposite sides of a cyclic quadrilateral (provided they are not parallel) intersect at right triangle.
In following fig., O is the centre of the circle. Find ∠ CBD.

In the figure, ∠DBC = 58°. BD is a diameter of the circle. Calculate : ∠BAC

In the given figure, AB is the diameter of a circle with centre O.
∠BCD = 130°. Find:
- ∠DAB
- ∠DBA
In the given circle with centre O, ∠ABC = 100°, ∠ACD = 40° and CT is a tangent to the circle at C. Find ∠ADC and ∠DCT.

