Advertisements
Advertisements
प्रश्न
In following fig., O is the centre of the circle. Find ∠ CBD.

उत्तर
It is given that ∠ AOC = 100°
Arc AC subtends ∠ AOC at the centre of circle and ∠ APC on the circumference of the circle
∴ ∠ AOC = 2 c
⇒ ∠ APC = `(100°)/2` = 50°
It can be seen that APCB is a cyclic quadriIateral.
∴ ∠ APC + ∠ ABC = 180° (Sum of opposite angles of a cyclic quadrilateral)
⇒ ∠ ABC = 180° - 50° = 130°
Now , ∠ ABC + ∠ CBD = 180° (Linear pair angles)
∠ CBD = 180° - 130° = 50°
APPEARS IN
संबंधित प्रश्न
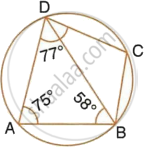
In the figure, given below, ABCD is a cyclic quadrilateral in which ∠BAD = 75°; ∠ABD = 58° and ∠ADC = 77°. Find:
- ∠BDC,
- ∠BCD,
- ∠BCA.
ABCD is a cyclic quadrilateral in which BC is parallel to AD, angle ADC = 110° and angle BAC = 50°. Find angle DAC and angle DCA.
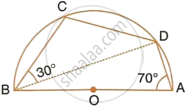
In the given figure, C and D are points on the semi-circle described on AB as diameter. Given angle BAD = 70° and angle DBC = 30°, calculate angle BDC.
ABCDE is a cyclic pentagon with centre of its circumcircle at point O such that AB = BC = CD and angle ABC = 120°.
Calculate:
- ∠BEC
- ∠BED
In the given figure, AB is parallel to DC, ∠BCE = 80° and ∠BAC = 25°.

Find:
- ∠CAD
- ∠CBD
- ∠ADC
In the given figure, AB is the diameter. The tangent at C meets AB produced at Q.

If ∠ CAB = 34° , find : ∠ CQB
In cyclic quadrilateral ABCD, ∠DAC = 27°; ∠DBA = 50° and ∠ADB = 33°. Calculate : ∠CAB.

Prove that the quadrilateral formed by angle bisectors of a cyclic quadrilateral ABCD is also cyclic.
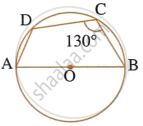
In the given figure, AB is the diameter of a circle with centre O.
∠BCD = 130°. Find:
- ∠DAB
- ∠DBA
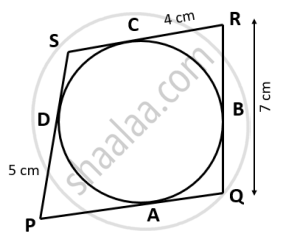
In the given figure, the sides of the quadrilateral PQRS touches the circle at A, B, C and D. If RC = 4 cm, RQ = 7 cm and PD = 5 cm. Find the length of PQ: