Advertisements
Advertisements
प्रश्न
ABCD is a cyclic quadrilateral in which BC is parallel to AD, angle ADC = 110° and angle BAC = 50°. Find angle DAC and angle DCA.
उत्तर
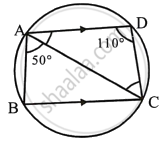
ABCD is a cyclic quadrilateral in which AD || BC
∠ADC = 110°, ∠BAC = 50°
∠B + ∠D = 180°
(Sum of opposite angles of a quadrilateral)
`=>` ∠B + 110° = 180°
`=>` ∠B = 70°
Now in ΔABC,
∠BAC + ∠ABC + ∠ACB = 180°
`=>` 50° + 70° + ∠ACB = 180°
`=>` ∠ACB = 180° – 120° = 60°
∵ AD || BC
∴ ∠DAC = ∠ACB = 60° ...(Alternate angles)
Now in ΔADC,
∠DAC + ∠ADC + ∠DCA = 180°
`=>` 60° + 110° + ∠DCA = 180°
`=>` ∠DCA = 180° – 170° = 10°
APPEARS IN
संबंधित प्रश्न
In the given figure, AB is the diameter of a circle with centre O. ∠BCD = 130o. Find:
1) ∠DAB
2) ∠DBA

The quadrilateral formed by angle bisectors of a cyclic quadrilateral is also cyclic. Prove it.
In the given figure, PAT is tangent to the circle with centre O at point A on its circumference and is parallel to chord BC. If CDQ is a line segment, show that:
- ∠BAP = ∠ADQ
- ∠AOB = 2∠ADQ
- ∠ADQ = ∠ADB
In a cyclic quadrilateral ABCD , AB || CD and ∠ B = 65° , find the remaining angles.
In following figure.,ABCD is a cyclic quadrilateral . If ∠ BCD = 100° and ∠ ABD = 70° , find ∠ ADB.
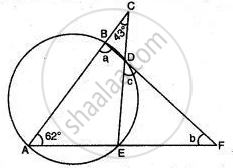
In the following figure, O is the centre of the circle. Find the values of a, b and c.

In the figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC. If angle AOB = 108°, find: ∠ADB.
In the given below figure,
∠ BAD = 65°
∠ ABD = 70°
∠ BDC = 45°
Find: (i) ∠ BCD, (ii) ∠ ADB.
Hence show that AC is a diameter.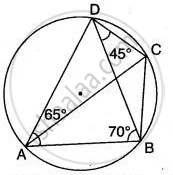
In ABCD is a cyclic quadrilateral; O is the centre of the circle. If BOD = 160°, find the measure of BPD.
In the given figure, if ∠ ACE = 43° and ∠CAF = 62°. Find the value of a, b, and c.