Advertisements
Advertisements
प्रश्न
In the given figure, AB is the diameter. The tangent at C meets AB produced at Q.

If ∠ CAB = 34° , find : ∠ CQB
उत्तर
QC is tangent to the circle
∴ ∠ CAB = ∠ QCB
Angle between tangent and chord = angle in alternate segment
∴ ∠ QCB = 34°
ABQ is a straight line
⇒ ∠ ABC + ∠ CBQ = 180°
⇒ 56° + ∠ CBQ = 180°
⇒ CBQ = 124°
Now,
∠ CQB = 180° - ∠ QCB = CBQ
⇒ ∠ CQB = 180° - 34°- 124°
⇒ ∠ CQB = 22°
APPEARS IN
संबंधित प्रश्न
In the given figure PQRS is a cyclic quadrilateral PQ and SR produced meet at T
1) Prove ΔTPS ~ ΔTRQ.
2) Find SP if TP = 18 cm, RQ = 4 cm and TR = 6 cm
3) Find the area of quadrilateral PQRS if the area of ΔPTS = 27 cm2.
In a cyclic quadrilateral ABCD, ∠A : ∠C = 3 : 1 and ∠B : ∠D = 1 : 5; find each angle of the quadrilateral.
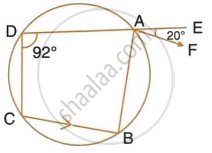
In the given figure, ABCD is a cyclic quadrilateral. AF is drawn parallel to CB and DA is produced to point E. If ∠ADC = 92°, ∠FAE = 20°; determine ∠BCD. Give reason in support of your answer.
In a cyclic quadrilateral ABCD , AB || CD and ∠ B = 65 ° , find the remaining angles

In a cyclic quadrilateral ABCD , AB || CD and ∠ B = 65° , find the remaining angles.
Bisectors of angles A, B and C of a triangle ABC intersect its circumcircle at D, E and F respectively. Prove that the angles of Δ DEF are 90° - `"A"/2` , 90° - `"B"/2` and 90° - `"C"/2` respectively.
In following figure.,ABCD is a cyclic quadrilateral . If ∠ BCD = 100° and ∠ ABD = 70° , find ∠ ADB.
ABCD is a parallelogram. A circle through vertices A and B meets side BC at point P and side AD at point Q. Show that quadrilateral PCDQ is cyclic.
In the figure, ∠DBC = 58°. BD is a diameter of the circle. Calculate : ∠BEC

In the given figure, the sides of the quadrilateral PQRS touches the circle at A, B, C and D. If RC = 4 cm, RQ = 7 cm and PD = 5 cm. Find the length of PQ: