Advertisements
Advertisements
प्रश्न
ABCD is a cyclic quadrilateral. Sides AB and DC produced meet at point E; whereas sides BC and AD produced meet at point F. If ∠DCF : ∠F : ∠E = 3 : 5 : 4, find the angles of the cyclic quadrilateral ABCD.
उत्तर
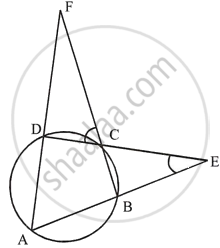
Given – In a circle, ABCD is a cyclic quadrilateral AB and DC are produced to meet at E and BC and AD are produced to meet at F.
∠DCF : ∠F : ∠E = 3 : 5 : 4
Let ∠DCF = 3x, ∠F = 5x, ∠E = 4x
Now, we have to find, ∠A, ∠B, ∠C and ∠D
In cyclic quad. ABCD, BC is produced.
∴ ∠A = ∠DCF = 3x
In ΔCDF,
Ext ∠CDA = ∠DCF + ∠F = 3x + 5x = 8x
In ΔBCE,
Ext ∠ABC = ∠BCE + ∠E ...[∠BCE = ∠DCF, Vertically opposite angles]
= ∠DCF + ∠E
= 3x + 4x
= 7x
Now, in cyclic quad ABCD,
Since, ∠B + ∠D = 180° ...[Since sum of opposite of a cyclic quadrilateral are supplementary]
`=>` 7x + 8x = 180°
`=>` 15x = 180°
`=> x = (180^circ)/15 = 12^circ`
∠A = 3x = 3 × 12° = 36°
∠B = 7x = 7 × 12° = 84°
∠C = 180° – ∠A = 180° – 36° = 144°
∠D = 8x = 8 × 12° = 96°
APPEARS IN
संबंधित प्रश्न
In the given figure, AB is the diameter of a circle with centre O. ∠BCD = 130o. Find:
1) ∠DAB
2) ∠DBA

In the given figure, AB = AC. Prove that DECB is an isosceles trapezium.
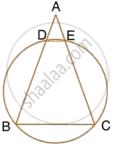
In the given figure, SP is bisector of ∠RPT and PQRS is a cyclic quadrilateral. Prove that : SQ = SR.

Bisectors of vertex angles A, B, and C of a triangle ABC intersect its circumcircle at the points D, E and F respectively. Prove that angle EDF = 90° – `1/2` ∠A.
In the given figure, AB is parallel to DC, ∠BCE = 80° and ∠BAC = 25°.

Find:
- ∠CAD
- ∠CBD
- ∠ADC
In following figure , O is the centre of the circle. If ∠ APB = 50° then find ∠ AOB and ∠ OAB.

In following figure.,ABCD is a cyclic quadrilateral . If ∠ BCD = 100° and ∠ ABD = 70° , find ∠ ADB.
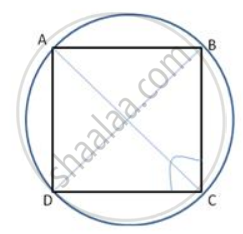
In following fig., O is the centre of the circle. Find ∠ CBD.

In the given below figure,
∠ BAD = 65°
∠ ABD = 70°
∠ BDC = 45°
Find: (i) ∠ BCD, (ii) ∠ ADB.
Hence show that AC is a diameter.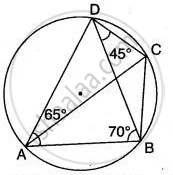
In the given figure O is the center of the circle, ∠ BAD = 75° and chord BC = chord CD. Find:
(i) ∠BOC (ii) ∠OBD (iii) ∠BCD.