Advertisements
Advertisements
प्रश्न
In the following figure, ABCD is a cyclic quadrilateral in which AD is parallel to BC.

If the bisector of angle A meets BC at point E and the given circle at point F, prove that:
- EF = FC
- BF = DF
उत्तर
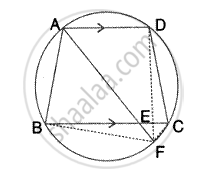
Given – ABCD is a cyclic quadrilateral in which AD || BC.
Bisector of ∠A meets BC at E and the given circle at F.
DF and BF are joined.
To prove –
- EF = FC
- BF = DF
Proof – ABCD is a cyclic quadrilateral and AD || BC
∵ AF is the bisector of ∠A, ∠BAF = ∠DAF
Also, ∠DAE = ∠BAE
∠DAE = ∠AEB ...[Alternate angles]
i. In ΔABE, ∠ABE = 180° – 2∠AEB
∠CEF = ∠AEB ...[Vertically opposite angles]
∠ADC = 180° – ∠ABC
= 180° – (180° – 2∠AEB)
∠ADC = 2∠AEB
∠AFC = 180° – ∠ADC
= 180° – 2∠AEB ...[Since ADFC is a cyclic quadrilateral]
∠ECF = 180° – (∠AFC + ∠CEF)
= 180° – (180° – 2∠AEB + ∠AEB)
= ∠AEB
∴ EF = FC
ii. ∴ Arc BF = Arc DF ...[Equal arcs subtends equal angles]
`=>` BF = DF ...[Equal arcs have equal chords]
Hence proved.
APPEARS IN
संबंधित प्रश्न
In cyclic quadrilateral ABCD, ∠DAC = 27°; ∠DBA = 50° and ∠ADB = 33°.
Calculate:
- ∠DBC,
- ∠DCB,
- ∠CAB.

In cyclic quadrilateral ABCD, ∠A = 3∠C and ∠D = 5∠B. Find the measure of each angle of the quadrilateral.
Bisectors of vertex angles A, B, and C of a triangle ABC intersect its circumcircle at the points D, E and F respectively. Prove that angle EDF = 90° – `1/2` ∠A.
In the given figure, ABCD is a cyclic quadrilateral, PQ is tangent to the circle at point C and BD is its diameter. If ∠DCQ = 40° and ∠ABD = 60°, find;
- ∠DBC
- ∠BCP
- ∠ADB
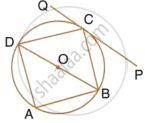
In a square ABCD, its diagonals AC and BD intersect each other at point O. The bisector of angle DAO meets BD at point M and the bisector of angle ABD meets AC at N and AM at L. Show that:
- ∠ONL + ∠OML = 180°
- ∠BAM + ∠BMA
- ALOB is a cyclic quadrilateral.
Two circles intersect in points P and Q. A secant passing through P intersects the circles in A and B respectively. Tangents to the circles at A and B intersect at T. Prove that A, Q, B and T lie on a circle.
In the given figure, AB is parallel to DC, ∠BCE = 80° and ∠BAC = 25°.

Find:
- ∠CAD
- ∠CBD
- ∠ADC
In the figure, ∠DBC = 58°. BD is a diameter of the circle. Calculate : ∠BEC

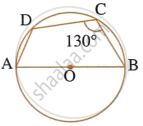
In the given figure, AB is the diameter of a circle with centre O.
∠BCD = 130°. Find:
- ∠DAB
- ∠DBA
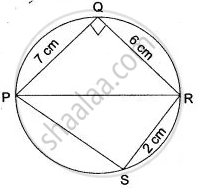
In the figure alongside PR is a diameter of the circle, PQ = 7 cm; QR = 6 cm and RS = 2 cm. Calculate the perimeter of the cyclic quadrilateral PQRS.