Advertisements
Advertisements
प्रश्न
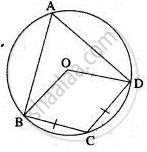
In the given figure O is the center of the circle, ∠ BAD = 75° and chord BC = chord CD. Find:
(i) ∠BOC (ii) ∠OBD (iii) ∠BCD.
उत्तर
(i) ∠ BOD = 2 x ∠ BAD = 2 x 75° = 150°
∠ BOC = ∠ COD
∵ BC = CD
∴ ∠ BOD = 2 ∠ BOC
∴ ∠ BOC = `1/2` ∠ BOD = 75°
(ii) ∠ OBD = `1/2`( 180° - ∠ BOD)
∠ OBD = `1/2`( 180° - 150°) = 15°
(iii) ∠ BCD = 180° - ∠ BAD ....(Opposite ∠S of a cyclic quadrilateral is supplementary.)
∠ BCD = 180° - 75°
∠ BCD = 105°
APPEARS IN
संबंधित प्रश्न
In a cyclic quadrilateral ABCD, ∠A : ∠C = 3 : 1 and ∠B : ∠D = 1 : 5; find each angle of the quadrilateral.
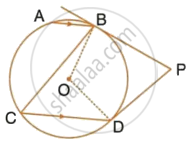
In the given figure, O is the centre of the circle. The tangents at B and D intersect each other at point P. If AB is parallel to CD and ∠ABC = 55°, find:
- ∠BOD
- ∠BPD
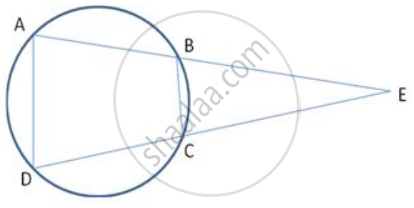
ABCD is a cyclic quadrilateral, AB and DC are produced to meet in E. Prove that ΔEBC ≅ ΔEDA.
In following fig., O is the centre of the circle. Find ∠ CBD.

ABCDE is a cyclic pentagon with centre of its circumcircle at point O such that AB = BC = CD and angle ABC=120°.
Calculate: ∠ BED.
In the following figure, O is the centre of the circle. Find the values of a, b and c.

In the figure, ∠DBC = 58°. BD is a diameter of the circle. Calculate : ∠BDC
In the given below the figure, O is the centre of the circle and ∠ AOC = 160°. Prove that 3∠y - 2∠x = 140°.

If O is the centre of the circle, find the value of x in each of the following figures

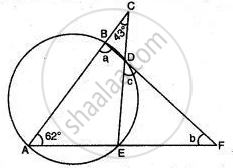
In the given figure, if ∠ ACE = 43° and ∠CAF = 62°. Find the value of a, b, and c.