Advertisements
Advertisements
प्रश्न
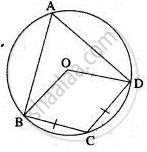
In the given figure O is the center of the circle, ∠ BAD = 75° and chord BC = chord CD. Find:
(i) ∠BOC (ii) ∠OBD (iii) ∠BCD.
उत्तर
(i) ∠ BOD = 2 x ∠ BAD = 2 x 75° = 150°
∠ BOC = ∠ COD
∵ BC = CD
∴ ∠ BOD = 2 ∠ BOC
∴ ∠ BOC = `1/2` ∠ BOD = 75°
(ii) ∠ OBD = `1/2`( 180° - ∠ BOD)
∠ OBD = `1/2`( 180° - 150°) = 15°
(iii) ∠ BCD = 180° - ∠ BAD ....(Opposite ∠S of a cyclic quadrilateral is supplementary.)
∠ BCD = 180° - 75°
∠ BCD = 105°
APPEARS IN
संबंधित प्रश्न
PQRS is a cyclic quadrilateral. Given ∠QPS = 73°, ∠PQS = 55° and ∠PSR = 82°, calculate:
1) ∠QRS
2) ∠RQS
3) ∠PRQ

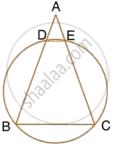
In the given figure, AB = AC. Prove that DECB is an isosceles trapezium.
In cyclic quadrilateral ABCD, ∠A = 3∠C and ∠D = 5∠B. Find the measure of each angle of the quadrilateral.
Use the given figure to find:
- ∠BAD,
- ∠DQB.
In a cyclic quadrilateral ABCD , AB || CD and ∠ B = 65° , find the remaining angles.
In a cyclic quadrialteral ABCD , if m ∠ A = 3 (m ∠C). Find m ∠ A.
In triangle ABC, AB = AC. A circle passing through B and c intersects the sides AB and AC at D and E respectively. Prove that DE || BC.
In the following figure, Prove that AD is parallel to FE.

Prove that the angle bisectors of the angles formed by producing opposite sides of a cyclic quadrilateral (Provided they are not parallel) intersect at the right angle.
In the figure , Δ PQR is an isosceles triangle with PQ = PR, and m ∠ PQR = 35°. Find m ∠ QSR and ∠ QTR.
