Advertisements
Advertisements
प्रश्न
In the given figure, O is the centre of the circle. Tangents at A and B meet at C. If ∠ACO = 30°,
find: (i) ∠ BCO (ii) ∠ AOB (iii) ∠ APB
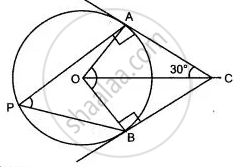
उत्तर
(i) ∠ BCO = ∠ ACO = 30° .....( ∴ C is the intersecting point of tangents AC and BC)
(ii) ∠ OAC = ∠ OBC = 90°
∠ ACO = 30° .....(Given)
∠ AOC = ∠ BOC = 180° - (90° + 30°) ....(Sum of the angles of a Δ is 180°)
∠ AOC = 180° - 120°
∠ AOC = 60°
∠ AOB = ∠ AOC + ∠ BOC
∠ AOB = 60° + 60° = 120°
(iii) ∠ APB =
APPEARS IN
संबंधित प्रश्न
Draw a circle and two lines parallel to a given line such that one is a tangent and the other, a secant to the circle.
Prove that the tangent drawn at the mid-point of an arc of a circle is parallel to the chord joining the end points of the arc.
The distance between two parallel tangents of a circle of radius 4 cm is ______
Two parallel lines touch the circle at
points A and B respectively. If the area of the circle is 25 n cm2, then AB is equal to ______
In the following figure, PA and PB are tangents from a point P to a circle with centre O. Then the quadrilateral OAPB must be a ______

A: What is a line called, if it meets the circle at only one point?
B: Collection of all points equidistant from a fixed point is ______.
- Chord
- Tangent
- Circle
- Curve
- Secant
Which is correct matching?
In the given figure, PT is a tangent to the circle at T, chord BA is produced to meet the tangent at P. Perpendicular BC bisects the chord TA at C. If PA = 9 cm and TB = 7 cm, find the lengths of:
- AB
- PT

The distance between two tangents parallel to each other of a circle is 13 cm. Find the radius of the circle.
In the given figure, PQ is a tangent to the circle with centre O. If ∠OPQ = x, ∠POQ = y, then x + y is ______.

In the given figure, PT is a tangent at T to the circle with centre O. If ∠TPO = 25°, then x is equal to ______.

