Advertisements
Advertisements
प्रश्न

In the given figure, QR is a common tangent to the two given circles touching externally at A. The tangent at A meets QR at P. If AP = 4.2 cm, then the length of QR is ______.
विकल्प
4.2 cm
2.1 cm
8.4 cm
6.3 cm
उत्तर
In the given figure, QR is a common tangent to the two given circles touching externally at A. The tangent at A meets QR at P. If AP = 4.2 cm, then the length of QR is 8.4 cm.
Explanation:
The figure shows two circles touching externally at point A, with QR being a common tangent. The tangent at A meets QR at point P, and we are given that the length AP = 4.2 cm. To find the length of QR, we can use the fact that the tangents drawn from an external point to a circle are equal in length.
Since A is the point of tangency for both circles, the lengths of the tangents from P to each point of tangency (which are Q and R respectively) will be equal. Thus, PQ = PR = AP.
Given AP = 4.2 cm, we can say PQ = PR = 4.2 cm.
Therefore, the length of QR,
QR = PQ + PR
QR = 4.2 + 4.2
QR = 8.4 cm
APPEARS IN
संबंधित प्रश्न
In the given figure, find TP if AT = 16 cm and AB = 12 cm.

In following fig., PT is a tangent to the circle at T and PAB is a secant to the same circle. If PB = 9cm and AB = Scm, find PT.
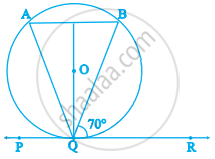
In figure, if PQR is the tangent to a circle at Q whose centre is O, AB is a chord parallel to PR and ∠BQR = 70°, then ∠AQB is equal to ______.
Tangents from an external point to a circle are ______
The quadrilateral formed by joining the angle bisectors of a cyclic quadrilateral is a ______
ABCD is a cyclic quadrilateral PQ is a tangent at B. If ∠DBQ = 65°, then ∠BCD is ______

Construct a pair of tangents to a circle of radius 5 cm which are inclined to each other at an angle of 60°.
In the given figure, PA is a tangent to the circle drawn from the external point P and PBC is the secant to the circle with BC as diameter. If ∠AOC = 130°, then find the measure of ∠APB, where O is the centre of the circle.

The length of tangent drawn to a circle of radius 9 cm from a point 41 cm from the centre is ______.
In the given figure O, is the centre of the circle. CE is a tangent to the circle at A. If ∠ABD = 26° find:
- ∠BDA
- ∠BAD
- ∠CAD
- ∠ODB

