Advertisements
Advertisements
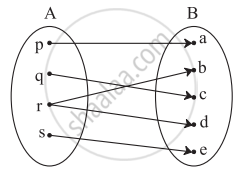
प्रश्न
Let A and B be any two sets such that n(B) = p, n(A) = q then the total number of functions f : A → B is equal to ______.
उत्तर
Let A and B be any two sets such that n(B) = p, n(A) = q then the total number of functions f : A → B is equal to pq functions.
Explanation:
Any element of set A
Say xi can be connected with the element of set B in p ways.
Hence, there are exactly pq functions.
APPEARS IN
संबंधित प्रश्न
Let f : R+ → R, where R+ is the set of all positive real numbers, such that f(x) = loge x. Determine
(c) whether f(xy) = f(x) : f(y) holds
The function f is defined by \[f\left( x \right) = \begin{cases}x^2 , & 0 \leq x \leq 3 \\ 3x, & 3 \leq x \leq 10\end{cases}\]
The relation g is defined by \[g\left( x \right) = \begin{cases}x^2 , & 0 \leq x \leq 2 \\ 3x, & 2 \leq x \leq 10\end{cases}\]
Show that f is a function and g is not a function.
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(iv) \[\frac{f}{g}\]
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(viii) \[\frac{5}{8}\]
The range of f(x) = cos [x], for π/2 < x < π/2 is
If 2f (x) − \[3f\left( \frac{1}{x} \right) = x^2\] (x ≠ 0), then f(2) is equal to
Let f(x) = x, \[g\left( x \right) = \frac{1}{x}\] and h(x) = f(x) g(x). Then, h(x) = 1
The domain of definition of the function \[f\left( x \right) = \sqrt{x - 1} + \sqrt{3 - x}\] is
The domain of definition of \[f\left( x \right) = \sqrt{x - 3 - 2\sqrt{x - 4}} - \sqrt{x - 3 + 2\sqrt{x - 4}}\] is
The range of the function \[f\left( x \right) = \frac{x}{\left| x \right|}\] is
If ƒ(m) = m2 − 3m + 1, find f(x + 1)
Which of the following relations are functions? If it is a function determine its domain and range:
{(1, 1), (3, 1), (5, 2)}
Check if the following relation is a function.
Express the following exponential equation in logarithmic form
`9^(3/2)` = 27
Express the following logarithmic equation in exponential form
`log_5 1/25` = – 2
Write the following expression as a single logarithm.
5 log x + 7 log y − log z
If f(x) = 3x + 5, g(x) = 6x − 1, then find (f + g) (x)
Answer the following:
Identify the following relation is the function? If it is a function determine its domain and range
{(12, 1), (3, 1), (5, 2)}
Answer the following:
A function f is defined as : f(x) = 5 – x for 0 ≤ x ≤ 4. Find the value of x such that f(x) = 5
Answer the following:
If f(x) = ax2 + bx + 2 and f(1) = 3, f(4) = 42, find a and b
Answer the following:
Simplify `log_10 28/45 - log_10 35/324 + log_10 325/432 - log_10 13/15`
Answer the following:
If `log ((x - y)/5) = 1/2 logx + 1/2 log y`, show that x2 + y2 = 27xy
Answer the following:
If a2 = b3 = c4 = d5, show that loga bcd = `47/30`
A function f is defined by f(x) = 2x – 3 find `("f"(0) + "f"(1))/2`
Let A = {1, 2, 3, 4} and B = N. Let f : A → B be defined by f(x) = x3 then, find the range of f
If a function f(x) is given as f(x) = x2 – 6x + 4 for all x ∈ R, then f(–3) = ______.
Let f : R → R be defined by
f(x) = `{(3x; x > 2),(2x^2; 1 ≤ x ≤ 2), (4x; x < 1):}`
Then f(-2) + f(1) + f(3) is ______
Find the range of the following functions given by `sqrt(16 - x^2)`
The domain of the function f given by f(x) = `(x^2 + 2x + 1)/(x^2 - x - 6)` is ______.
The domain and range of the function f given by f(x) = 2 – |x – 5| is ______.
