Advertisements
Advertisements
प्रश्न
Line PQ is parallel to line RS where points P,Q,R and S have
co-ordinates (2, 4), (3, 6), (3, 1) and (5, k) respectively. Find value of k.
उत्तर
slope of the line =`(y_2-y_1)/(x_2-x_1)`
P(2, 4), Q(3, 6)
slope of the line PQ = `(6-4)/(3-2) = 2/1 = 2`
R
(3, 1), S (5, k)
slope of the line RS = `(k−1)/(5-3) = (k−1)/2`
But line PQ || line RS
∴ slope of line PQ = slope of line RS
∴ `2 = (k−1)/2`
∴ 4 = k - 1
∴ 4 + 1 = k
∴ k = 5
APPEARS IN
संबंधित प्रश्न
In ΔABC, A(3, 5), B(7, 8) and C(1, –10). Find the equation of the median through A.
Given 3x + 2y + 4 = 0
(i) express the equation in the form y = mx + c
(ii) Find the slope and y-intercept of the line 3x + 2y + 4 = 0
Find the slope and y-intercept of the line:
y = 4
The equation of a line is x – y = 4. Find its slope and y-intercept. Also, find its inclination.
Is the line 3x + 2y = 5 parallel to the line x + 2y = 1?
Find the equation of the line passing through (−2, 1) and perpendicular to 4x + 5y = 6.
Find the equation of the perpendicular bisector of the line segment obtained on joining the points (6, −3) and (0, 3).
A = (7, −2) and C = (−1, −6) are the vertices of square ABCD. Find the equations of diagonals AC and BD.
- Write down the equation of the line AB, through (3, 2) and perpendicular to the line 2y = 3x + 5.
- AB meets the x-axis at A and the y-axis at B. Write down the co-ordinates of A and B. Calculate the area of triangle OAB, where O is the origin.
The line 4x − 3y + 12 = 0 meets x-axis at A. Write the co-ordinates of A. Determine the equation of the line through A and perpendicular to 4x – 3y + 12 = 0.
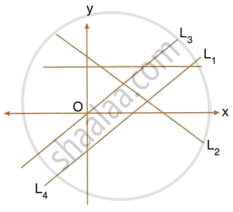
Match the equations A, B, C and D with the lines L1, L2, L3 and L4, whose graphs are roughly drawn in the given diagram.
A ≡ y = 2x;
B ≡ y – 2x + 2 = 0;
C ≡ 3x + 2y = 6;
D ≡ y = 2
Find the equation of the line which is perpendicular to the line `x/a - y/b = 1` at the point where this line meets y-axis.
Find the point on the X–axis which is equidistant from A(–3, 4) and B(1, –4).
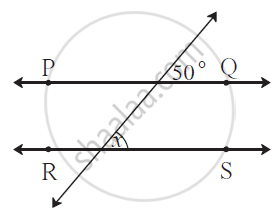
In the figure, line PQ || line RS. Using the information given
in the figure find the value of x.
Prove that :
“If a line parallel to a side of a triangle intersects the remaining sides in two distince points, then the line divides the sides in the same proportion.”
Find Equation of CD

A line through point P(4, 3) meets x-axis at point A and the y-axis at point B. If BP is double of PA, find the equation of AB.
Find the equation of the line through the points A(–1, 3) and B(0, 2). Hence, show that the point A, B and C(1, 1) are collinear.
A line is parallel to Y-axis and is at a distance of 5 units from the Y-axis. Write the equation of that line.
