Advertisements
Advertisements
प्रश्न
PQRS is a square with each side 6cm. T is a point on QR such that the `"area of ΔPQT"/"area of trapezium PTRS" = (1)/(3)` Find the length of TR.
उत्तर
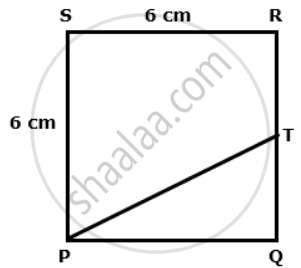
`"Area of ΔPQT"/"Area of trapezium PTRS" = (1)/(3)`
⇒ `((1)/(2) xx "PQ" xx "QT")/((1)/(2) xx ("TR" + "SP") xx "RS") = (1)/(3)`
⇒ `("PQ" xx ("QR" - "TR"))/(("TR" + "SP") xx "RS") = (1)/(3)`
⇒ `(6 xx (6 - "TR"))/(("TR" + 6) xx 6) = (1)/(3)`
⇒ `(36 - 6"TR")/("TR" + 6)` = 2
⇒ 36 - 6TR = 2TR + 12
⇒ 8TR = 24
⇒ TR = 3cm.
APPEARS IN
संबंधित प्रश्न
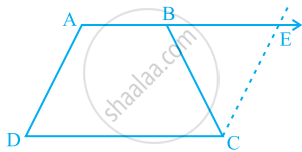
ABCD is a trapezium in which AB || CD and AD = BC (see the given figure). Show that
- ∠A = ∠B
- ∠C = ∠D
- ΔABC ≅ ΔBAD
- diagonal AC = diagonal BD
[Hint: Extend AB and draw a line through C parallel to DA intersecting AB produced at E.]
State, 'true' or 'false'
The quadrilateral, whose four sides are equal, is a square.
In the figure, given below, AM bisects angle A and DM bisects angle D of parallelogram ABCD. Prove that: ∠AMD = 90°.

ABCD is a parallelogram having an area of 60cm2. P is a point on CD. Calculate the area of ΔAPB.
In the figure, PT is parallel to SR. QTSR is a parallelogram and PQSR is a rectangle. If the area of ΔQTS is 60cm2, find:
(i) the area o || gm QTSR
(ii) the area of the rectangle PQRS
(iii) the area of the triangle PQS.
In the given figure area of ∥ gm PQRS is 30 cm2. Find the height of ∥ gm PQFE if PQ = 6 cm.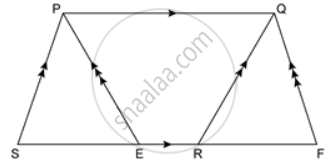
The diagonals of a parallelogram ABCD intersect at O. A line through O meets AB in P and CD in Q. Show that
(a) Area of APQD = `(1)/(2)` area of || gm ABCD
(b) Area of APQD = Area of BPQC
Prove that the median of a triangle divides it into two triangles of equal area.
Find the area of each of the following figure:
Find the area of quadrilateral, whose diagonals of lengths 18 cm and 13 cm intersect each other at right angle.
One side of a parallelogram is 12cm and the altitude corresponding to i is 8cm. If the length of the altitude corresponding to its adjacent side is 16cm, find the length of the adjacent side.
A rectangular field 240m long has an area 36000m2. Find the cost of fencing the field at Rs.2.50per m.
The area of a square garden is equal to the area of a rectangular plot of length 160m and width 40m. Calculate the cost of fencing the square garden at Rs.12per m.
The area of a square plot of side 80m is equal to the area of a rectangular plot of length 160m. Calculate the width of the rectangular plot and the cost of fencing it Rs.7.50per m.
Find the area of a rhombus whose perimeter is 260cm and the length of one of its diagonal is 66cm.
A footpath of uniform width runs all around the inside of a rectangular garden of 40 m x 30 m. If the path occupies 136 m2, find the width of the path.
The diagonals of a square are perpendicular to one another.
Give reason for the following :
A square can be thought of as a special rectangle.
Give reasons for the following :
A square can be thought of as a special rhombus.
Examine whether the following is a polygon. If it is not, say why?