Question

Prove that the lines joining the middle points of the opposite sides of a quadrilateral and the join of the middle points of its diagonals meet in a point and bisect one another

Answer 1

Let us consider a Cartesian plane having a parallelogram OABC in which O is the origin.

We have to prove that middle point of the opposite sides of a quadrilateral and the join of the mid-points of its diagonals meet in a point and bisect each other. 

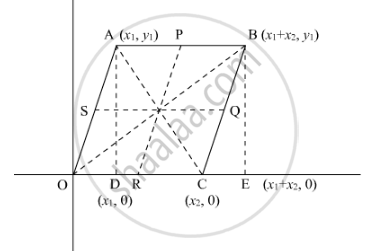
Let the co-ordinate of A be `(x_1,y_1)`. So the coordinates of other vertices of the quadrilateral are- O (0, 0); B`(x_1+x_2,y_1)`; C`(x_2,0)` 

 

Let P, Q, R and S be the mid-points of the sides AB, BC, CD, DA respectively.

In general to find the mid-point p(x,y)  of two points`A(x_1,y_-1)`and B`(x_2,y_2)` we use section formula as, 

`P(x,y)=((x_1+x_2)/2,(y_1+y_2)/2)` 

So co-ordinate of point P, 

`=((x_1+x_2+x_1)/2,(y_1+y_-2)/2)` 

`=((2x_1+x_1)/2,y_1/2)` 

Similarly co-ordinate of point R,

`=(x_2/2,0)` 

Similary co-ordinate of point S, 

`=(x_1/2,y_1/2)`

Let us find the co-ordinates of mid-point of as, 

`(((2x_1+x_2)/2+x_2/2)/2,y_1/2)` 

`=((x_1+x_2)/ 2,y_1/2)` 

Similarly co-ordinates of mid-point of Qs, 

`=((x_1+x_2)/2,y_1/2)` 

NOw the mid-pont of diagonal AC, 

`=((x_1+x_2)/2,y_1/2)` 

Similarly the mid-point of diagonal OA, 

`((x_1+x_2)/2,y_1/2)` 

Hence the mid-points of PR, QS, AC and OA coincide.

Thus, middle point of the opposite sides of a quadrilateral and the join of the mid-points of its diagonals meet in a point and bisect each other.