Advertisements
Advertisements
प्रश्न
Ratio of two adjacent sides of a parallelogram is 3 : 4, and its perimeter is 112 cm. Find the length of its each side.
उत्तर
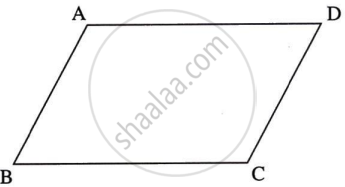
Let `square`ABCD be a parallelogram.
AB : BC = 3 : 4
If AB = 3x cm
So, BC = 4x cm ...(x is the common multiple.)
∴ CD = AB = 3x cm and AD = BC = 4x cm ...(opposite sides of parallelogram)
Perimeter of `square`ABCD = 112 cm
∴ AB + BC + CD + AD = 112
∴ 3x + 4x + 3x + 4x = 112
∴ 14x = 112
∴ x = `112/14`
∴ x = 8
∴ CD = AB = 3x = 3 × 8 = 24 cm
∴ AD = BC = 4x = 4 × 8 = 32 cm
APPEARS IN
संबंधित प्रश्न
Consider the given parallelogram. Find the values of the unknowns x, y, z.

The measures of two adjacent angles of a parallelogram are in the ratio 3 : 2. Find the measure of each of the angles of the parallelogram.
The following figure GUNS is a parallelogram. Find x and y. (Lengths are in cm)

Name the quadrilaterals whose diagonals bisect each other
Referring the adjacent figure of a parallelogram, write the answer of questions given below.

(1) If l(WZ) = 4.5 cm then l(XY) = ?
(2) If l(YZ) = 8.2 cm then l(XW) = ?
(3) If l(OX) = 2.5 cm then l(OZ) = ?
(4) If l(WO) = 3.3 cm then l(WY) = ?
(5) If m∠WZY = 120° then m∠WXY = ? and m∠XWZ = ?
In parallelogram ABCD, E is the mid-point of side AB and CE bisects angle BCD. Prove that :
(i) AE = AD,
(ii) DE bisects and ∠ADC and
(iii) Angle DEC is a right angle.
In the following diagram, the bisectors of interior angles of the parallelogram PQRS enclose a quadrilateral ABCD.
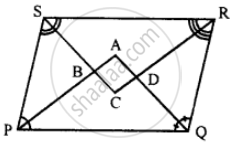
Show that:
(i) ∠PSB + ∠SPB = 90°
(ii) ∠PBS = 90°
(iii) ∠ABC = 90°
(iv) ∠ADC = 90°
(v) ∠A = 90°
(vi) ABCD is a rectangle
Thus, the bisectors of the angles of a parallelogram enclose a rectangle.
If two adjacent angles of a parallelogram are (5x – 5)° and (10x + 35)°, then the ratio of these angles is ______.
A diagonal of a parallelogram bisects an angle. Will it also bisect the other angle? Give reason.
Construct a parallelogram ABCD in which AB = 4 cm, BC = 5 cm and ∠B = 60°.
