Advertisements
Advertisements
प्रश्न
Solve the following inequation and represent the solution set on a number line.
`-8 1/2 < -1/2 -4x <= 7 1/2`, x ∈ 1
उत्तर
`-81/2 < -1/2-4x<=71/2`, x ∈ I
`=> -17/2 < -1/2 - 4x <= 15/2`, x ∈ I
Take `-17/2 < -1/2 - 4x` `-1/2 - 4x <= 15/2`
`-17/2 + 1/2 < -4x` `-4x <= 15/2 + 1/2`
`-16/2 < -4x` `-4x <= 16/2`
-8 < -4x `-4x <= 8`
2 > x `x >= -2`
Thus, on simplifying, the given inequation reduces to -2 ≤ x 2.
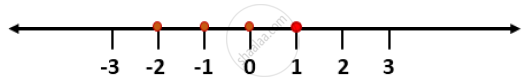
Since x ∈ I, the solution set is { -2, -1, 0, 1}.
The required graph on the number line is as follows:
APPEARS IN
संबंधित प्रश्न
State, true or false :
The line `x/2 + y/3 = 0` passes through the point (4, −6).
Find the equation of the line passing through : (0, 1) and (1, 2)
A, B and C have co-ordinates (0, 3), (4, 4) and (8, 0) respectively. Find the equation of the line through A and perpendicular to BC.
The line through P (5, 3) intersects y-axis at Q.
write the equation of the line
Find if the following points lie on the given line or not:
(5,3) on the line 3x - 5y + 5 = 0
Find the value of a line parallel to the following line:
`(3"y")/4 + (5"y")/2 = 7`
Find the value of a line parallel to the following line:
`(2"x")/5 + "y"/3` = 2
Find the equation of a line passing through (8,3) and making an angle of 45° with the positive direction of the y-axis.
Find the equation of a line passing through (2,9) and parallel to the line 3x + 4y = 11
Find the equation of the straight line perpendicular to 5x – 2y = 8 and which passes through the mid-point of the line segment joining (2, 3) and (4, 5).
