Advertisements
Advertisements
प्रश्न
The conductivity of 0.20 mol L−1 solution of KCl is 2.48 × 10−2 S cm−1. Calculate its molar conductivity and degree of dissociation (α). Given λ0 (K+) = 73.5 S cm2 mol−1 and λ0 (C1−) = 76.5 S cm2 mol−1.
Advertisements
उत्तर
Conductivity of KCl solution = 2.48×10–2 S cm–1
Concentration of KCl solution = 0.20 mol L–1
= 0.20 × 1000 mol cm–3
= 200 mol cm–3
Molar conductivity
`Lambda_m=k/c`
`=(2.48xx10^(-2)" S "cm^(-1))/(200" mol "cm^(-3))`
`=124xx10^(-6)" S "cm^2" mol"^(-1)`
Given:
`lambda_(K^+)^@=73.5" S "cm^2" mol"^(-1)`
`lambda_(Cl^-)^@=76.5" S "cm^2" mol"^(-1)`
Degree of dissociation
`alpha=Lambda_m/Lambda_m^@" "" "" "" ".......("i")`
`Lambda_(m(KCl))^@=lambda_(K+)^@+lambda_(Cl^-)^@`
`=73.5+76.5`
`=150" S "cm^2" mol"^(-1)`
`"Substituting the values of "Lambda_m" and "Lambda_(m(KCl))^@" in (i), we get"`
`alpha=(124xx10^(-6)" S "cm^2" mol"^(-1))/(150" S "cm^2" mol"^(-1))=0.82xx10^(-6)`
संबंधित प्रश्न
State Kohlrausch’s law of independent migration of ions.
The conductivity of 0.20 M solution of KCl at 298 K is 0.025 S cm−1. Calculate its molar conductivity.
Define the following terms: Molar conductivity (⋀m)
The molar conductivity of 0.025 mol L−1 methanoic acid is 46.1 S cm2 mol−1. Calculate its degree of dissociation and dissociation constant. Given \[\ce{λ^0_{(H^+)}}\] = 349.6 S cm2 mol−1 and \[\ce{λ^0_{(HCOO^-)}}\] = 54.6 S cm2 mol−1.
In the plot of molar conductivity (∧m) vs square root of concentration (c1/2) following curves are obtained for two electrolytes A and B : 
Answer the following:
(i) predict the nature of electrolytes A and B.
(ii) What happens on the extrapolation of ∧m to concentration approaching for electrolytes A and B?
Assertion: `"E"_("Ag"^+ //"Ag")` increases with increase in concentration of Ag+ ions.
Reason: `"E"_("Ag"^+ //"Ag")` has a positive value.
Consider figure and answer the question to given below.
How will the concentration of Zn2+ ions and Ag+ ions be affected after the cell becomes ‘dead’?
Given below are two statements:
Statements I: The limiting molar conductivity of KCl (strong electrolyte) is higher compared to that of CH3COOH (weak electrolyte).
Statement II: Molar conductivity decreases with decrease in concentration of electrolyte.
In the light of the above statements, choose the most appropriate answer from the options given below:
The solubility of Co2[Fe(CN)6] in water at 25°C from the following data:
Conductivity of saturated solution of Co2[Fe(CN)6] = 2.06 × 10−6 ohm−1 cm−1 and that of water = 4.1 × 10−7 ohm−1 cm−1. The ionic molar conductivities of Co2+ and [Fe(CN)6]4− are 86 and 444 ohm−1 cm2 mol−1 respectively, is ______ × 10−6 mol/L.
The variation of molar conductivity with concentration of an electrolyte (X) m aqueous solution is shown in the given figure.
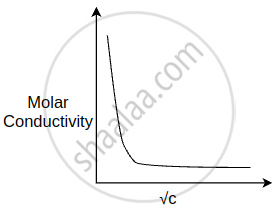
The electrolyte X is ______.
