Advertisements
Advertisements
प्रश्न
The ear-ring of a lady shown in figure has a 3 cm long light suspension wire. (a) Find the time period of small oscillations if the lady is standing on the ground. (b) The lady now sits in a merry-go-round moving at 4 m/s1 in a circle of radius 2 m. Find the time period of small oscillations of the ear-ring.

उत्तर
Given,
Length of the long, light suspension wire, l = 3 cm = 0.03 m
Acceleration due to gravity, `g = 9.8 "ms"^(- 2)`
(a)Time Period \[\left( T \right)\] is given by ,
\[T = 2\pi\sqrt{\left( \frac{l}{g} \right)}\]
\[ = 2\pi\sqrt{\left( \frac{0 . 03}{9 . 8} \right)}\]
\[ = 0 . 34 \text { second}\]
(b) Velocity of merry-go-round, v = 4 `"ms"^(- 1)`
Radius of circle, r = 2 m
As the lady sits on the merry-go-round, her earring experiences centripetal acceleration.
Centripetal acceleration (a) is given by,
\[a = \frac{v^2}{r} = \frac{4^2}{2} = 8 m/ s^2\]
Resultant acceleration (A) is given by ,
\[A = \sqrt{\left( g^2 + a^2 \right)}\]
\[ = \sqrt{\left( 96 . 04 + 64 \right)}\]
\[ = 12 . 65 m/ s^2\]
Time Period,
\[T = 2\pi\sqrt{\left( \frac{l}{A} \right)}\]
\[= 2\pi\sqrt{\left( \frac{0 . 03}{12 . 65} \right)}\]
\[ = 0 . 30 \text { second }\]
APPEARS IN
संबंधित प्रश्न
A copper metal cube has each side of length 1 m. The bottom edge of the cube is fixed and tangential force 4.2x108 N is applied to a top surface. Calculate the lateral displacement of the top surface if modulus of rigidity of copper is 14x1010 N/m2.
Which of the following example represent (nearly) simple harmonic motion and which represent periodic but not simple harmonic motion?
General vibrations of a polyatomic molecule about its equilibrium position.
Answer in brief:
Derive an expression for the period of motion of a simple pendulum. On which factors does it depend?
Two bodies A and B of equal mass are suspended from two separate massless springs of spring constant k1 and k2 respectively. If the bodies oscillate vertically such that their maximum velocities are equal, the ratio of the amplitude of A to that of B is
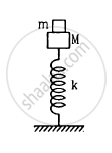
A small block of mass m is kept on a bigger block of mass M which is attached to a vertical spring of spring constant k as shown in the figure. The system oscillates vertically. (a) Find the resultant force on the smaller block when it is displaced through a distance x above its equilibrium position. (b) Find the normal force on the smaller block at this position. When is this force smallest in magnitude? (c) What can be the maximum amplitude with which the two blocks may oscillate together?
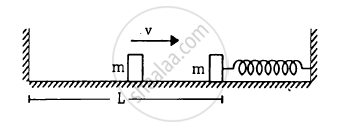
The left block in figure moves at a speed v towards the right block placed in equilibrium. All collisions to take place are elastic and the surfaces are frictionless. Show that the motions of the two blocks are periodic. Find the time period of these periodic motions. Neglect the widths of the blocks.
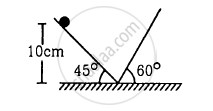
Find the time period of the motion of the particle shown in figure . Neglect the small effect of the bend near the bottom.
The period of oscillation of a body of mass m1 suspended from a light spring is T. When a body of mass m2 is tied to the first body and the system is made to oscillate, the period is 2T. Compare the masses m1 and m2
A 20 cm wide thin circular disc of mass 200 g is suspended to rigid support from a thin metallic string. By holding the rim of the disc, the string is twisted through 60° and released. It now performs angular oscillations of period 1 second. Calculate the maximum restoring torque generated in the string under undamped conditions. (π3 ≈ 31)
Find the number of oscillations performed per minute by a magnet is vibrating in the plane of a uniform field of 1.6 × 10-5 Wb/m2. The magnet has a moment of inertia 3 × 10-6 kg/m2 and magnetic moment 3 A m2.
The maximum speed of a particle executing S.H.M. is 10 m/s and maximum acceleration is 31.4 m/s2. Its periodic time is ______
Which of the following example represent periodic motion?
A freely suspended bar magnet displaced from its N-S direction and released.
Which of the following example represent (nearly) simple harmonic motion and which represent periodic but not simple harmonic motion?
The motion of a ball bearing inside a smooth curved bowl, when released from a point slightly above the lowermost point.
When two displacements represented by y1 = a sin(ωt) and y2 = b cos(ωt) are superimposed the motion is ______.
Show that the motion of a particle represented by y = sin ωt – cos ωt is simple harmonic with a period of 2π/ω.
The time period of a simple pendulum is T inside a lift when the lift is stationary. If the lift moves upwards with an acceleration `g/2`, the time period of the pendulum will be ______.
When a particle executes Simple Harmonic Motion, the nature of the graph of velocity as a function of displacement will be ______.
