Advertisements
Advertisements
प्रश्न
The equation of a travelling sound wave is y = 6.0 sin (600 t − 1.8 x) where y is measured in 10−5 m, t in second and x in metre. (a) Find the ratio of the displacement amplitude of the particles to the wavelength of the wave. (b) Find the ratio of the velocity amplitude of the particles to the wave speed.
उत्तर
Given:
Equation of a travelling sound wave is y = 6.0 sin (600 t − 1.8 x),
where y is measured in 10−5 m,
t in second,
x in metre.
Comparing the given equation with the wave equation, we find:
Amplitude A = 6 \[\times\]10-5 m
\[(a) \text{ We have: } \]
\[ \frac{2\pi}{\lambda} = 1 . 8 \]
\[ \Rightarrow \lambda = \left( \frac{2\pi}{1 . 8} \right)\]
\[\text { So, required ratio: } \]
\[ \frac{A}{\lambda} = \frac{6 . 0 \times (1 . 8) \times {10}^{- 5} m/s}{(2\pi)} = 1 . 7 \times {10}^{- 5} m\]
(b) Let Vy be the velocity amplitude of the wave.
\[\text { Velocity v }= \frac{dy}{dt}\]
\[v = \frac{d\left[ 6 \sin \left( 600 t - 1 . 8 x \right) \right]}{dt}\]
\[ \Rightarrow v = 3600 \cos (600t - 1 . 8x) \times {10}^{- 5} m/s\]
\[\text { Amplitute } V_y = 3600 \times {10}^{- 5} m/s\]
\[\text { Wavelength: }\]
\[ \lambda = \frac{2\pi}{1 . 8}\]
\[\text { Time period: } \]
\[T = \frac{2\pi}{\omega}\]
\[ \Rightarrow T = \frac{2\pi}{600}\]
\[\text { Wave speed v } = \frac{\lambda}{T}\]
\[ \Rightarrow v = \frac{600}{1 . 8} = \frac{100}{3} m/s\]
\[\text { Required ratio: } \]
\[\left( \frac{V_y}{v} \right) = \frac{3600 \times 3 \times {10}^{- 5}}{1000} = 1 . 1 \times {10}^{- 4} m\]
APPEARS IN
संबंधित प्रश्न
The wavelengths of two sound waves in air are `81/173`m and `81/170`m. They produce 10 beats per second. Calculate the velocity of sound in air
What is the smallest positive phase constant which is equivalent to 7⋅5 π?
Can you hear your own words if you are standing in a perfect vacuum? Can you hear your friend in the same conditions?
Two tuning forks vibrate with the same amplitude but the frequency of the first is double the frequency of the second. Which fork produces more intense sound in air?
When two waves with same frequency and constant phase difference interfere,
When you speak to your friend, which of the following parameters have a unique value in the sound produced?
A source of sound moves towards an observer.
A listener is at rest with respect to the source of sound. A wind starts blowing along the line joining the source and the observer. Which of the following quantities do not change?
(a) Frequency
(b) Velocity of sound
(c) Wavelength
(d) Time period
A sound wave frequency 100 Hz is travelling in air. The speed of sound in air is 350 m s−1. (a) By how much is the phase changed at a given point in 2.5 ms? (b) What is the phase difference at a given instant between two points separated by a distance of 10.0 cm along the direction of propagation?
At what temperature will the speed of sound be double of its value at 0°C?
The absolute temperature of air in a region linearly increases from T1 to T2 in a space of width d. Find the time taken by a sound wave to go through the region in terms of T1, T2, d and the speed v of sound at 273 K. Evaluate this time for T1 = 280 K, T2 = 310 K, d = 33 m and v = 330 m s−1.
The intensity of sound from a point source is 1.0 × 10−8 W m−2 at a distance of 5.0 m from the source. What will be the intensity at a distance of 25 m from the source?
Sound with intensity larger than 120 dB appears pain full to a person. A small speaker delivers 2.0 W of audio output. How close can the person get to the speaker without hurting his ears?
A string, fixed at both ends, vibrates in a resonant mode with a separation of 2⋅0 cm between the consecutive nodes. For the next higher resonant frequency, this separation is reduced to 1⋅6 cm. Find the length of the string.
Three sources of sound S1, S2 and S3 of equal intensity are placed in a straight line with S1S2 = S2S3. At a point P, far away from the sources, the wave coming from S2 is 120° ahead in phase of that from S1. Also, the wave coming from S3 is 120° ahead of that from S2. What would be the resultant intensity of sound at P?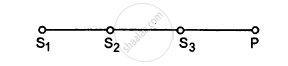
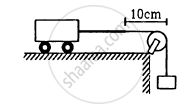
A heavy string is tied at one end to a movable support and to a light thread at the other end as shown in following figure. The thread goes over a fixed pulley and supports a weight to produce a tension. The lowest frequency with which the heavy string resonates is 120 Hz. If the movable support is pushed to the right by 10 cm so that the joint is placed on the pulley, what will be the minimum frequency at which the heavy string can resonate?
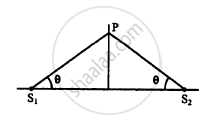
Two sources of sound S1 and S2 vibrate at same frequency and are in phase. The intensity of sound detected at a point P as shown in the figure is I0. (a) If θ equals 45°, what will be the intensity of sound detected at this point if one of the sources is switched off? (b) What will be the answer of the previous part if θ = 60°?
The separation between a node and the next antinode in a vibrating air column is 25 cm. If the speed of sound in air is 340 m s−1, find the frequency of vibration of the air column.
A small source of sound oscillates in simple harmonic motion with an amplitude of 17 cm. A detector is placed along the line of motion of the source. The source emits a sound of frequency 800 Hz which travels at a speed of 340 m s−1. If the width of the frequency band detected by the detector is 8 Hz, find the time period of the source.
A source of sound emitting a 1200 Hz note travels along a straight line at a speed of 170 m s−1. A detector is placed at a distance 200 m from the line of motion of the source. (a) Find the frequency of sound receive by the detector at the instant when the source gets closest to it. (b) Find the distance between the source and the detector at the instant in detects the frequency 1200 Hz. Velocity of sound in air = 340 m s−1.
