Advertisements
Advertisements
प्रश्न
The line `x/a + y/b` = 1 moves in such a way that `1/a^2 + 1/b^2 = 1/c^2`, where c is a constant. The locus of the foot of the perpendicular from the origin on the given line is x2 + y2 = c2.
विकल्प
True
False
उत्तर
This statement is True.
Explanation:
The given equation is `x/b - y/a` = 0 .......(i)
Equation of the line passing through (0, 0) and perpendicular to equation (i) is
`x/b - y/a` = 0 .....(ii)
Squaring and adding equation (i) and (ii) we get
`(x/a + y/b)^2 + (x/b - y/a)^2` = 1 + 0
⇒ `x^2/a^2 + y^2/b^2 + (2xy)/(ab) + x^2/b^2 + y^2/a^2 - (2xy)/(ab)` = 1
⇒ `x^2(1/a^2 + 1/b^2) + y^2(1/b^2 + 1/a^2)` = 1
⇒ `(x^2 + y^2) (1/a^2 + 1/b^2)` = 1
⇒ `(x^2 + y^2)(1/c^2)` = 1 ....`[because 1/a^2 + 1/b^2 = 1/c^2]`
⇒ x2 + y2 = c2
APPEARS IN
संबंधित प्रश्न
Find the distance between P (x1, y1) and Q (x2, y2) when :
- PQ is parallel to the y-axis,
- PQ is parallel to the x-axis
Find the equation of a line drawn perpendicular to the line `x/4 + y/6 = 1`through the point, where it meets the y-axis.
Find the equation of the lines through the point (3, 2) which make an angle of 45° with the line x –2y = 3.
Find the slope of a line passing through the following point:
(−3, 2) and (1, 4)
What is the value of y so that the line through (3, y) and (2, 7) is parallel to the line through (−1, 4) and (0, 6)?
What can be said regarding a line if its slope is zero ?
What can be said regarding a line if its slope is positive ?
If three points A (h, 0), P (a, b) and B (0, k) lie on a line, show that: \[\frac{a}{h} + \frac{b}{k} = 1\].
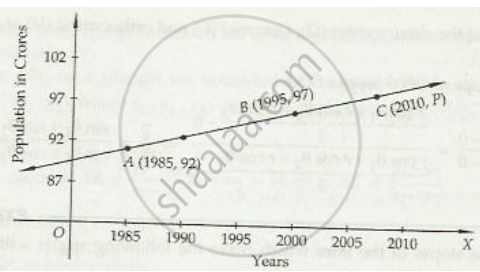
Consider the following population and year graph:
Find the slope of the line AB and using it, find what will be the population in the year 2010.
Find the angle between the X-axis and the line joining the points (3, −1) and (4, −2).
Find the value of x for which the points (x, −1), (2, 1) and (4, 5) are collinear.
A quadrilateral has vertices (4, 1), (1, 7), (−6, 0) and (−1, −9). Show that the mid-points of the sides of this quadrilateral form a parallelogram.
Find the equation of a straight line with slope 2 and y-intercept 3 .
Find the equations of the bisectors of the angles between the coordinate axes.
Find the equation of the right bisector of the line segment joining the points (3, 4) and (−1, 2).
Find the angles between the following pair of straight lines:
3x + y + 12 = 0 and x + 2y − 1 = 0
Find the angles between the following pair of straight lines:
x − 4y = 3 and 6x − y = 11
Find the angles between the following pair of straight lines:
(m2 − mn) y = (mn + n2) x + n3 and (mn + m2) y = (mn − n2) x + m3.
Prove that the points (2, −1), (0, 2), (2, 3) and (4, 0) are the coordinates of the vertices of a parallelogram and find the angle between its diagonals.
If two opposite vertices of a square are (1, 2) and (5, 8), find the coordinates of its other two vertices and the equations of its sides.
If the slope of a line passing through the point A(3, 2) is `3/4`, then find points on the line which are 5 units away from the point A.
The two lines ax + by = c and a′x + b′y = c′ are perpendicular if ______.
The equation of the line passing through (1, 2) and perpendicular to x + y + 7 = 0 is ______.
Show that the tangent of an angle between the lines `x/a + y/b` = 1 and `x/a - y/b` = 1 is `(2ab)/(a^2 - b^2)`
Find the equation of a straight line on which length of perpendicular from the origin is four units and the line makes an angle of 120° with the positive direction of x-axis.
Find the equation of one of the sides of an isosceles right angled triangle whose hypotenuse is given by 3x + 4y = 4 and the opposite vertex of the hypotenuse is (2, 2).
Slope of a line which cuts off intercepts of equal lengths on the axes is ______.
The point (4, 1) undergoes the following two successive transformations:
(i) Reflection about the line y = x
(ii) Translation through a distance 2 units along the positive x-axis Then the final coordinates of the point are ______.
The vertex of an equilateral triangle is (2, 3) and the equation of the opposite side is x + y = 2. Then the other two sides are y – 3 = `(2 +- sqrt(3)) (x - 2)`.
