Advertisements
Advertisements
प्रश्न
The ratio between the altitudes of two similar triangles is 3 : 5; write the ratio between their :
- corresponding medians.
- perimeters.
- areas.
उत्तर
In ΔABC ∼ ΔPQR and AL : PM = 3 : 5
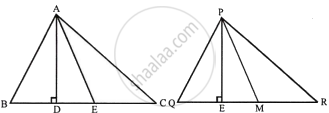
Now, we have to find the ratio between their.
- corresponding medians
- perimeters and
- areas.
AD and PE are the medians of ΔABC and ΔPQR respectively.
∵ ΔABC ∼ ΔPQR
∴ ∠B = ∠Q and `(AB)/(PQ) = (BC)/(QR)`
Now in ΔABL and PQM,
∠B = ∠Q ...(Proved)
∠L = ∠M ...(Each 90°)
i. ∴ ΔABL ∼ ΔPQM
∴ `(AB)/(PQ) = (AL)/(PM) = 3/5` (Given) ...(i)
∵ ΔABC ∼ ΔPQR
∴ `(AB)/(DE) = (BC)/(QR) = (2BD)/(2QE) = (BD)/(QE)`
And ∠B = ∠Q
∴ ΔABD ∼ ΔPQE
∴ `(AB)/(PQ) = (AD)/(PE) = 3/5` ...[From (i)]
∴ `(AD)/(PE) = 3 : 5`
ii. ∵ ΔABC ∼ ΔPQR
∴ `(AB)/(PQ) = (BC)/(QR) = (CA)/(RP)`
= `(AB + BC + CA)/(PQ + QR + RP)`
= `3/5` ...[From (i)]
Hence ratio between their perimeters = 3 : 5
iii. ∵ ΔABC ∼ ΔPQR
∴ `(Area ΔABC)/(Area ΔPQR) = (AB^2)/(PQ^2) = (3)^2/(5)^2 = 9/25`
∴ The ratio between their areas = 9 : 25
APPEARS IN
संबंधित प्रश्न
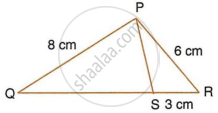
PQR is a triangle. S is a point on the side QR of ΔPQR such that ∠PSR = ∠QPR. Given QP = 8 cm, PR = 6 cm and SR = 3 cm.
- Prove ΔPQR ∼ ΔSPR.
- Find the length of QR and PS.
- `"area of ΔPQR"/"area of ΔSPR"`
In ∆ABC, right – angled at C, CD ⊥ AB.
Prove:
`"CD"^2 = "AD"xx "DB"`
In the given figure, AX : XB = 3 : 5
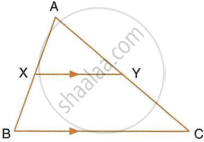
Find:
- the length of BC, if the length of XY is 18 cm.
- the ratio between the areas of trapezium XBCY and triangle ABC.
In the figure, given below, ABCD is a parallelogram. P is a point on BC such that BP : PC = 1 : 2. DP produced meets AB produces at Q. Given the area of triangle CPQ = 20 cm2.

Calculate:
- area of triangle CDP,
- area of parallelogram ABCD.
In the given figure, ∠B = ∠E, ∠ACD = ∠BCE, AB = 10.4 cm and DE = 7.8 cm. Find the ratio between areas of the ∆ABC and ∆DEC.
The dimensions of the model of a multistoreyed building are 1 m by 60 cm by 1.20 m. If the scale factor is 1 : 50, find the actual dimensions of the building.
Also, find:
- the floor area of a room of the building, if the floor area of the corresponding room in the model is 50 sq. cm.
- the space (volume) inside a room of the model, if the space inside the corresponding room of the building is 90 m3.
Two isosceles triangles have equal vertical angles. Show that the triangles are similar. If the ratio between the areas of these two triangles is 16 : 25, find the ratio between their corresponding altitudes.
In triangle ABC, AP : PB = 2 : 3. PO is parallel to BC and is extended to Q so that CQ is parallel to BA.
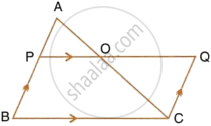
Find:
- area ΔAPO : area ΔABC.
- area ΔAPO : area ΔCQO.
In the given figure, ABC is a right angled triangle with ∠BAC = 90°.
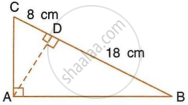
- Prove that : ΔADB ∼ ΔCDA.
- If BD = 18 cm and CD = 8 cm, find AD.
- Find the ratio of the area of ΔADB is to area of ΔCDA.
In fig. ABCD is a trapezium in which AB | | DC and AB = 2DC. Determine the ratio between the areas of ΔAOB and ΔCOD.