Advertisements
Advertisements
Question
The ratio between the altitudes of two similar triangles is 3 : 5; write the ratio between their :
- corresponding medians.
- perimeters.
- areas.
Solution
In ΔABC ∼ ΔPQR and AL : PM = 3 : 5
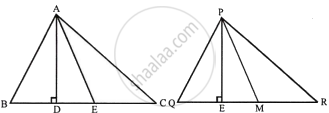
Now, we have to find the ratio between their.
- corresponding medians
- perimeters and
- areas.
AD and PE are the medians of ΔABC and ΔPQR respectively.
∵ ΔABC ∼ ΔPQR
∴ ∠B = ∠Q and `(AB)/(PQ) = (BC)/(QR)`
Now in ΔABL and PQM,
∠B = ∠Q ...(Proved)
∠L = ∠M ...(Each 90°)
i. ∴ ΔABL ∼ ΔPQM
∴ `(AB)/(PQ) = (AL)/(PM) = 3/5` (Given) ...(i)
∵ ΔABC ∼ ΔPQR
∴ `(AB)/(DE) = (BC)/(QR) = (2BD)/(2QE) = (BD)/(QE)`
And ∠B = ∠Q
∴ ΔABD ∼ ΔPQE
∴ `(AB)/(PQ) = (AD)/(PE) = 3/5` ...[From (i)]
∴ `(AD)/(PE) = 3 : 5`
ii. ∵ ΔABC ∼ ΔPQR
∴ `(AB)/(PQ) = (BC)/(QR) = (CA)/(RP)`
= `(AB + BC + CA)/(PQ + QR + RP)`
= `3/5` ...[From (i)]
Hence ratio between their perimeters = 3 : 5
iii. ∵ ΔABC ∼ ΔPQR
∴ `(Area ΔABC)/(Area ΔPQR) = (AB^2)/(PQ^2) = (3)^2/(5)^2 = 9/25`
∴ The ratio between their areas = 9 : 25
APPEARS IN
RELATED QUESTIONS
Given: ABCD is a rhombus, DPR and CBR are straight lines.
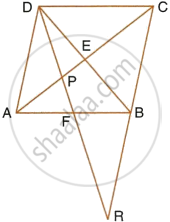
Prove that: DP × CR = DC × PR.
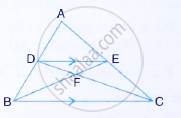
Given : AB || DE and BC || EF. Prove that :
- `(AD)/(DG) = (CF)/(FG)`
- ∆DFG ∼ ∆ACG
In the given figure, ABC is a triangle. DE is parallel to BC and `(AD)/(DB)=3/2`
(1) Determine the ratios `(AD)/(AB) and (DE)/(BC)`
(2 ) Prove that ∆DEF is similar to ∆CBF Hence, find `(EF)/(FB)`.
(3) What is the ratio of the areas of ∆DEF and ∆BFC.
Triangle ABC is an isosceles triangle in which AB = AC = 13 cm and BC = 10 cm. AD is
perpendicular to BC. If CE = 8 cm and EF ⊥ AB, find:
i)`"area of ADC"/"area of FEB"` ii)`"area of ΔAFEB"/"area of ΔABC"`
In the given figure, triangle ABC is similar to triangle PQR. AM and PN are altitudes whereas AX and PY are medians.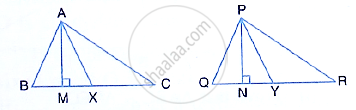
Prove that : `(AM)/(PN)=(AX)/(PY)`
The ratio between the areas of two similar triangles is 16 : 25. State the ratio between their :
- perimeters.
- corresponding altitudes.
- corresponding medians.
In the following figure, AB, CD and EF are parallel lines. AB = 6cm, CD = y cm, EF = 10 cm, AC = 4 cm and CF = x cm. Calculate x and y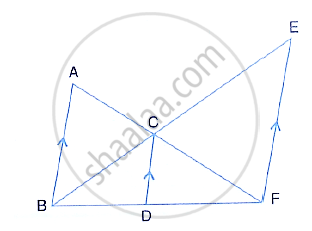 
In ΔABC, ∠ACB = 90° and CD ⊥ AB.
Prove that : `(BC^2)/(AC^2)=(BD)/(AD)`
In triangle ABC, AP : PB = 2 : 3. PO is parallel to BC and is extended to Q so that CQ is parallel to BA.
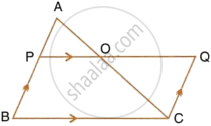
Find:
- area ΔAPO : area ΔABC.
- area ΔAPO : area ΔCQO.
In the give figure, ABC is a triangle with ∠EDB = ∠ACB. Prove that ΔABC ∼ ΔEBD. If BE = 6 cm, EC = 4 cm, BD = 5 cm and area of ΔBED = 9 cm2. Calculate the:
- length of AB
- area of ΔABC
