Advertisements
Advertisements
Question
In triangle ABC, AP : PB = 2 : 3. PO is parallel to BC and is extended to Q so that CQ is parallel to BA.
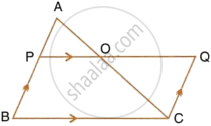
Find:
- area ΔAPO : area ΔABC.
- area ΔAPO : area ΔCQO.
Solution
In ΔABC,
AP : PB = 2 : 3
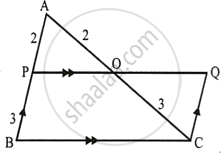
PQ || BC and CQ || BA
∵ PQ || BC
∴ `(AP)/(PB) = (AO)/(OC) = 2/3`
i. In ΔAPQ ∼ ΔABC
∴ `(Area ΔAPO)/(Area ΔABC) = (AP^2)/(AB^2)`
= `(AP^2)/(AP + PB)^2`
= `(2)^2/(2 + 3)^2`
= `4/25`
∴ area ΔAPO : area ΔABC = 4 : 25
ii. In ΔAPO and ΔCQO
∠APO = ∠OQC ...(Alternate angles)
∠AOP = ∠COQ ...(Vertically opposite angles)
∴ ΔAPO ∼ ΔCQO ...(AA axiom)
∴ `(Area ΔAPO)/(Area ΔCQO) = (AP^2)/(CQ^2)`
= `(AP^2)/(PB^2)` {∵ PBCQ is a || gm}
= `(2)^2/(3)^2`
= `4/9`
∴ area ΔAPO : area ΔCQO = 4 : 9
APPEARS IN
RELATED QUESTIONS
In ∆ ABC, ∠B = 2 ∠C and the bisector of angle B meets CA at point D. Prove that:
(i) ∆ ABC and ∆ ABD are similar,
(ii) DC: AD = BC: AB
In the given triangle PQR, LM is parallel to QR and PM : MR = 3 : 4.
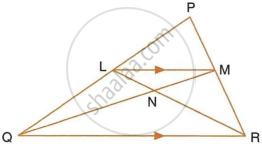
Calculate the value of ratio:
- `(PL)/(PQ)` and then `(LM)/(QR)`
- `"Area of ΔLMN"/"Area of ΔMNR"`
- `"Area of ΔLQM"/"Area of ΔLQN"`
Triangle ABC is an isosceles triangle in which AB = AC = 13 cm and BC = 10 cm. AD is
perpendicular to BC. If CE = 8 cm and EF ⊥ AB, find:
i)`"area of ADC"/"area of FEB"` ii)`"area of ΔAFEB"/"area of ΔABC"`

An aeroplane is 30 m long and its model is 15 cm long. If the total outer surface area of the model is 150 cm2, find the cost of painting the outer surface of the aeroplane at the rate of Rs.120 per sq. m. Given that 50 sq. m of the surface of the aeroplane is left for windows.
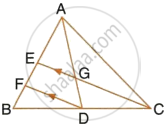
In the following figure, AD and CE are medians of ΔABC. DF is drawn parallel to CE. Prove that :
- EF = FB,
- AG : GD = 2 : 1
In the given figure, triangle ABC is similar to triangle PQR. AM and PN are altitudes whereas AX and PY are medians.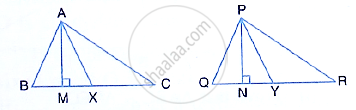 Prove that : `(AM)/(PN)=(AX)/(PY)`
Prove that : `(AM)/(PN)=(AX)/(PY)`
In the following figure, AB, CD and EF are parallel lines. AB = 6cm, CD = y cm, EF = 10 cm, AC = 4 cm and CF = x cm. Calculate x and y 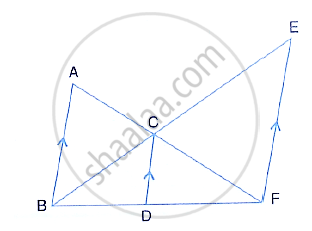
In ΔABC, ∠ACB = 90° and CD ⊥ AB.
Prove that : `(BC^2)/(AC^2)=(BD)/(AD)`
A triangle ABC with AB = 3 cm, BC = 6 cm and AC = 4 cm is enlarged to ΔDEF such that the longest side of ΔDEF = 9 cm. Find the scale factor and hence, the lengths of the other sides of ΔDEF.
Two isosceles triangles have equal vertical angles. Show that the triangles are similar. If the ratio between the areas of these two triangles is 16 : 25, find the ratio between their corresponding altitudes.
