Advertisements
Advertisements
प्रश्न
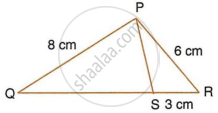
PQR is a triangle. S is a point on the side QR of ΔPQR such that ∠PSR = ∠QPR. Given QP = 8 cm, PR = 6 cm and SR = 3 cm.
- Prove ΔPQR ∼ ΔSPR.
- Find the length of QR and PS.
- `"area of ΔPQR"/"area of ΔSPR"`
उत्तर
i. In ΔPQR and ΔSPR, we have
∠QPR = ∠PSR ...(Given)
∠PRQ = ∠PRS ...(Common)
So, by AA-axiom similarity, we have
ΔPQR ∼ ΔSPR ...(Proved)
ii. Since ΔPQR ∼ ΔSPR ...(Proved)
`=> (PQ)/(SP) = (QR)/(PR) = (PR)/(SR)`
Consider `(QR)/(PR) = (PR)/(SR)` ...(From 1)
`=> (QR)/6 = 6/3`
`=> QR = (6 xx 6)/3 = 12 cm`
Also, `(PQ)/(SP) = (PR)/(SR)`
`=> 8/(SP) = 6/3`
`=>8/(SP) = 2`
`=> SP = 8/2 = 4 cm`
iii. `"area of ΔPQR"/"area of ΔSPR" = (PQ^2)/(SP^2)`
= `8^2/4^2`
= `64/16`
= `4/1`
`"area of ΔPQR"/"area of ΔSPR" = 4 : 1`
APPEARS IN
संबंधित प्रश्न
In ∆ABC, ∠B = 90° and BD ⊥ AC.
- If CD = 10 cm and BD = 8 cm; find AD.
- If AC = 18 cm and AD = 6 cm; find BD.
- If AC = 9 cm and AB = 7 cm; find AD.
In the right-angled triangle QPR, PM is an altitude.
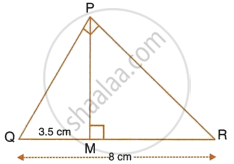
Given that QR = 8 cm and MQ = 3.5 cm, calculate the value of PR.
In the given triangle PQR, LM is parallel to QR and PM : MR = 3 : 4.
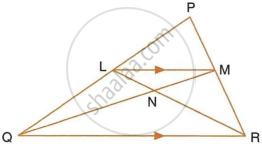
Calculate the value of ratio:
- `(PL)/(PQ)` and then `(LM)/(QR)`
- `"Area of ΔLMN"/"Area of ΔMNR"`
- `"Area of ΔLQM"/"Area of ΔLQN"`
In the figure, given below, ABCD is a parallelogram. P is a point on BC such that BP : PC = 1 : 2. DP produced meets AB produces at Q. Given the area of triangle CPQ = 20 cm2.

Calculate:
- area of triangle CDP,
- area of parallelogram ABCD.
In the given figure, ∠B = ∠E, ∠ACD = ∠BCE, AB = 10.4 cm and DE = 7.8 cm. Find the ratio between areas of the ∆ABC and ∆DEC.
In the given figure, triangle ABC is similar to triangle PQR. AM and PN are altitudes whereas AX and PY are medians.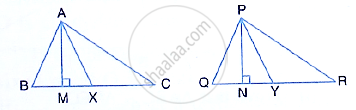 Prove that : `(AM)/(PN)=(AX)/(PY)`
Prove that : `(AM)/(PN)=(AX)/(PY)`
The ratio between the altitudes of two similar triangles is 3 : 5; write the ratio between their :
- corresponding medians.
- perimeters.
- areas.
On a map, drawn to a scale of 1 : 20000, a rectangular plot of land ABCD has AB = 24 cm and BC = 32 cm. Calculate :
- the diagonal distance of the plot in kilometer.
- the area of the plot in sq. km.
Two isosceles triangles have equal vertical angles. Show that the triangles are similar. If the ratio between the areas of these two triangles is 16 : 25, find the ratio between their corresponding altitudes.
In fig. ABCD is a trapezium in which AB | | DC and AB = 2DC. Determine the ratio between the areas of ΔAOB and ΔCOD.