Advertisements
Advertisements
प्रश्न
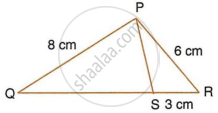
PQR is a triangle. S is a point on the side QR of ΔPQR such that ∠PSR = ∠QPR. Given QP = 8 cm, PR = 6 cm and SR = 3 cm.
- Prove ΔPQR ∼ ΔSPR.
- Find the length of QR and PS.
- `"area of ΔPQR"/"area of ΔSPR"`
उत्तर
i. In ΔPQR and ΔSPR, we have
∠QPR = ∠PSR ...(Given)
∠PRQ = ∠PRS ...(Common)
So, by AA-axiom similarity, we have
ΔPQR ∼ ΔSPR ...(Proved)
ii. Since ΔPQR ∼ ΔSPR ...(Proved)
`=> (PQ)/(SP) = (QR)/(PR) = (PR)/(SR)`
Consider `(QR)/(PR) = (PR)/(SR)` ...(From 1)
`=> (QR)/6 = 6/3`
`=> QR = (6 xx 6)/3 = 12 cm`
Also, `(PQ)/(SP) = (PR)/(SR)`
`=> 8/(SP) = 6/3`
`=>8/(SP) = 2`
`=> SP = 8/2 = 4 cm`
iii. `"area of ΔPQR"/"area of ΔSPR" = (PQ^2)/(SP^2)`
= `8^2/4^2`
= `64/16`
= `4/1`
`"area of ΔPQR"/"area of ΔSPR" = 4 : 1`
APPEARS IN
संबंधित प्रश्न
Given: ABCD is a rhombus, DPR and CBR are straight lines.
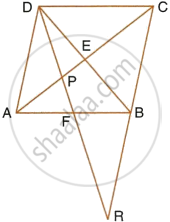
Prove that: DP × CR = DC × PR.
In ∆ABC, ∠B = 90° and BD ⊥ AC.
- If CD = 10 cm and BD = 8 cm; find AD.
- If AC = 18 cm and AD = 6 cm; find BD.
- If AC = 9 cm and AB = 7 cm; find AD.
In the given figure, AX : XB = 3 : 5
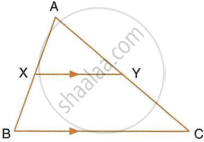
Find:
- the length of BC, if the length of XY is 18 cm.
- the ratio between the areas of trapezium XBCY and triangle ABC.
In the given figure, ABC is a triangle. DE is parallel to BC and `(AD)/(DB)=3/2`
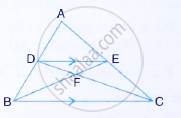
(1) Determine the ratios `(AD)/(AB) and (DE)/(BC)`
(2 ) Prove that ∆DEF is similar to ∆CBF Hence, find `(EF)/(FB)`.
(3) What is the ratio of the areas of ∆DEF and ∆BFC.
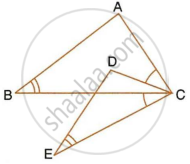
In the given figure, ∠B = ∠E, ∠ACD = ∠BCE, AB = 10.4 cm and DE = 7.8 cm. Find the ratio between areas of the ∆ABC and ∆DEC.
An aeroplane is 30 m long and its model is 15 cm long. If the total outer surface area of the model is 150 cm2, find the cost of painting the outer surface of the aeroplane at the rate of Rs.120 per sq. m. Given that 50 sq. m of the surface of the aeroplane is left for windows.
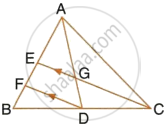
In the following figure, AD and CE are medians of ΔABC. DF is drawn parallel to CE. Prove that :
- EF = FB,
- AG : GD = 2 : 1
The ratio between the areas of two similar triangles is 16 : 25. State the ratio between their :
- perimeters.
- corresponding altitudes.
- corresponding medians.
In the following figure, AB, CD and EF are parallel lines. AB = 6cm, CD = y cm, EF = 10 cm, AC = 4 cm and CF = x cm. Calculate x and y 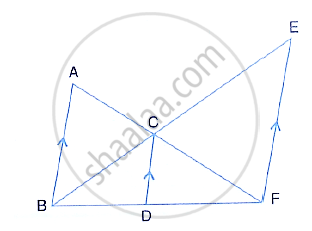
The dimensions of the model of a multistoreyed building are 1 m by 60 cm by 1.20 m. If the scale factor is 1 : 50, find the actual dimensions of the building.
Also, find:
- the floor area of a room of the building, if the floor area of the corresponding room in the model is 50 sq. cm.
- the space (volume) inside a room of the model, if the space inside the corresponding room of the building is 90 m3.
