Advertisements
Advertisements
प्रश्न
The sum of the length, breadth and height of a cuboid is `6sqrt(3)` cm and the length of its diagonal is `2sqrt(3)` cm. The total surface area of the cuboid is ______.
विकल्प
48 cm2
72 cm2
96 cm2
108 cm2
उत्तर
The sum of the length, breadth and height of a cuboid is `6sqrt(3)` cm and the length of its diagonal is `2sqrt(3)` cm. The total surface area of the cuboid is `underline(bb(96 cm^2)`.
Explanation:
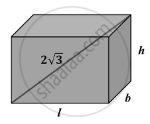
Let Length = l
Breadth = b
Height = h
Given that
Sum of the length, breadth and height of a cuboid is `6sqrt(3)` cm
l + b + h = `6sqrt(3)` cm ......(1)
Also, given that
Length of its diagonal is `2sqrt(3)` cm
`sqrt(l^2 + b^2 + h^2) = 2sqrt(3)` ......(2)
We need to find
Total surface area of the cuboid
Now, Total surface area of cuboid = 2(lb + bh + lh)
From (1)
l + b + h = `6sqrt(3)` cm
Squaring both sides
(l + b + h)2 = `(6sqrt(3))^2`
l2 + b2 + h2 + 2lb + 2bh + 2lh = `6^2 xx (sqrt(3))^2`
l2 + b2 + h2 + 2lb + 2bh + 2lh = 36 × 3
l2 + b2 + h2 + 2lb + 2bh + 2lh = 108
From (1)
`sqrt(l^2 + b^2 + h^2) = 2sqrt(3)`
Squaring both sides
`(sqrt(l^2 + b^2 + h^2))^2 = (2sqrt(3))^2`
l2 + b2 + h2 = 22 × `(sqrt(3))^2`
l2 + b2 + h2 = 4 × 3
l2 + b2 + h2 = 12
12 + 2lb + 2bh + 2lh = 108
2lb + 2bh + 2lh = 108 – 12
2lb + 2bh + 2lh = 96
Total surface area = 96 cm2
APPEARS IN
संबंधित प्रश्न
The largest sphere is to be curved out of a right circular of radius 7cm and height 14cm. find volume of sphere?
A hemispherical depression is cut out from one face of a cubical wooden block of edge 21 cm, such that the diameter of the hemisphere is equal to the edge of the cube. Determine the volume and total surface area of the remaining block.
A bucket made up of a metal sheet is in form of a frustum of cone of height 16cm with diameters of its lower and upper ends as 16cm and 40cm. find the volume of bucket. Also find cost of bucket if the cost of metal sheet used is Rs 20 per 100 cm2
Twelve solid spheres of the same size are made by melting a solid metallic cylinder of base diameter 2 cm and height 16 cm. The diameter of each sphere is ______.
How many bags of grain can be stored in a cuboidal granary (8 m × 6 m × 3 m), if each bag occupies a space of 0.64 m3?
Match the following columns:
| Column I | Column II |
| (a) A solid metallic sphere of radius 8 cm is melted and the material is used to make solid right cones with height 4 cm and base radius of 8 cm. How many cones are formed? | (p) 18 |
| (b) A 20-m-deep well with diameter 14 m is dug up and the earth from digging is evenly spread out to form a platform 44 m by 14 m. The height of the platform is ...........m. |
(q) 8 |
| (c) A sphere of radius 6 cm is melted and recast in the shape of a cylinder of radius 4 cm. Then, the height of the cylinder is ......... cm. |
(r) 16 : 9 |
| (d) The volumes of two spheres are in the ratio 64 : 27. The ratio of their surface areas is ....... . |
(s) 5 |
A hemispherical bowl of internal radius 9 cm is full of water. This water is to be filled in cylindrical bottles of diameter 3 cm and height 4 cm. Find the number of bottles needed in which the water can be filled.
Water in a canal, 6 m wide and 1.5 m deep, is flowing with a speed of 10 km/hour. How much area will it irrigate in 30 mins; if 8 cm standing water is needed?
A surahi is the combination of ______.
How many spherical lead shots each of diameter 4.2 cm can be obtained from a solid rectangular lead piece with dimensions 66 cm, 42 cm and 21 cm.
