Advertisements
Advertisements
प्रश्न
Two lines AB and CD intersect at O such that BC is equal and parallel to AD. Prove that the lines AB and CD bisect at O.
उत्तर
It is given that
BC = AD
BC || AD
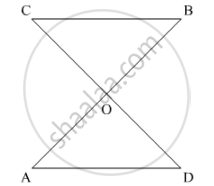
We have to prove that AB and CD bisect at O.
If we prove that ΔAOD ≅ ΔBOC , then
We can prove AB and CDbisects atO.
Now in ΔAOD and ΔBOC
AD = BC(Given)
∠OBC =∠OAD (Since AD || BC and AB is transversal)
And ∠OCB = ∠ODA(since AD || BC and CD is transversal)
So by ASAcongruence criterion we have,
ΔAOD ≅ ΔBOC, so
OA = OB
OD = OC
Hence ABand CD bisect each other at O.
APPEARS IN
संबंधित प्रश्न
Find the measure of each exterior angle of an equilateral triangle.
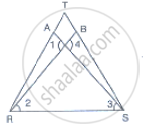
In Fig. 10.40, it is given that RT = TS, ∠1 = 2∠2 and ∠4 = 2∠3. Prove that ΔRBT ≅ ΔSAT
ABC is a triangle in which ∠B = 2 ∠C. D is a point on BC such that AD bisects ∠BAC and AB = CD.
Prove that ∠BAC = 72°.
Fill the blank in the following so that the following statement is true.
If altitudes CE and BF of a triangle ABC are equal, then AB = ....
Fill in the blank to make the following statement true.
The sum of any two sides of a triangle is .... than the third side.
Fill in the blank to make the following statement true.
If two angles of a triangle are unequal, then the smaller angle has the........ side opposite to it.
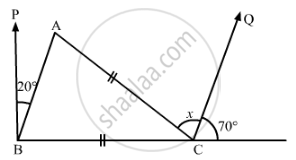
In the given figure, if BP || CQ and AC = BC, then the measure of x is
In the given figure, if l1 || l2, the value of x is

AD is a median of the triangle ABC. Is it true that AB + BC + CA > 2AD? Give reason for your answer.
Is it possible to construct a triangle with lengths of its sides as 8 cm, 7 cm and 4 cm? Give reason for your answer.
