Advertisements
Advertisements
प्रश्न
In the given figure, if BP || CQ and AC = BC, then the measure of x is
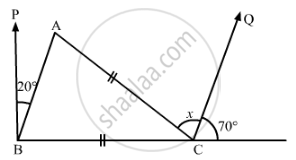
विकल्प
20°
25°
30°
35°
उत्तर
In the given figure, BP || CQ and AC || BC
We need to find the measure of x

Here, we draw a line RS parallel to BP, i.e BP || RS
Also, using the property, “two lines parallel to the same line are parallel to each other”
As,
BP || RS
BP || CQ
Thus, RS || CQ
Now, BP || RS and BA is the transversal, so using the property, “alternate interior angles are equal”
∠PBA = ∠BAS
∠BAS = 20° .......... (1)
Similarly, CQ|| RS and AC is the transversal
∠QCA = ∠SAC
∠SAC = x ........(2)
Adding (1) and (2), we get
∠SAC + ∠BAS = 20° + x
∠A = 20° + x
Also, as AC = BC
Using the property,”angles opposite to equal sides are equal”, we get
∠ABC = ∠CAB
∠ABC = 20° + x
Further, using the property, “an exterior angle is equal to the sum of the two opposite interior angles”
In ΔABC
ext. ∠C = ∠CAB + ∠ABC
70° + x = 20° + x + 20° + x
70° + x = 40° + 2x
70° - 40° = 2x - x
x = 30°
Thus, x = 30°
APPEARS IN
संबंधित प्रश्न
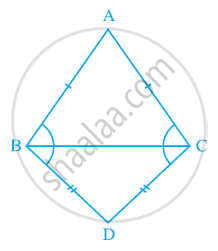
ABC and DBC are two isosceles triangles on the same base BC (see the given figure). Show that ∠ABD = ∠ACD.
If the bisector of the exterior vertical angle of a triangle be parallel to the base. Show that the triangle is isosceles.
In an isosceles triangle, if the vertex angle is twice the sum of the base angles, calculate the angles of the triangle.
In a ΔABC, it is given that AB = AC and the bisectors of ∠B and ∠C intersect at O. If M is a point on BO produced, prove that ∠MOC = ∠ABC.
ABC is a triangle in which ∠B = 2 ∠C. D is a point on BC such that AD bisects ∠BAC and AB = CD.
Prove that ∠BAC = 72°.
Fill in the blank to make the following statement true.
In a right triangle the hypotenuse is the .... side.
Line segments AB and CD intersect at O such that AC || DB. If ∠CAB = 45° and ∠CDB = 55°, then ∠BOD =
In the given figure, if EC || AB, ∠ECD = 70° and ∠BDO = 20°, then ∠OBD is
In ∆PQR, ∠R = ∠P and QR = 4 cm and PR = 5 cm. Then the length of PQ is ______.
Bisectors of the angles B and C of an isosceles triangle ABC with AB = AC intersect each other at O. Show that external angle adjacent to ∠ABC is equal to ∠BOC
