Advertisements
Advertisements
प्रश्न
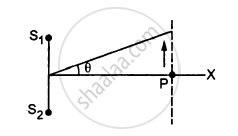
Two speakers S1 and S2, driven by the same amplifier, are placed at y = 1.0 m and y = −1.0 m(See figure). The speakers vibrate in phase at 600 Hz. A man stands at a point on the X-axis at a very large distance from the origin and starts moving parallel to the Y-axis. The speed of sound in air is 330 m s−1. (a) At what angle θ will the intensity of sound drop to a minimum for the first time? (b) At what angle will he hear a maximum of sound intensity for the first time? (c) If he continues to walk along the line, how many more can he hear?
उत्तर
Given :
Frequency of source f = 600 Hz
Speed of sound in air v = 330 m/s
\[v = f\lambda\]
\[\therefore \lambda = \frac{v}{f}\]=\[\frac{330}{600} = 0 . 5 \text { mm }\]
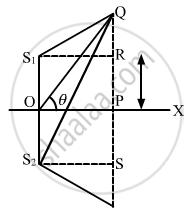
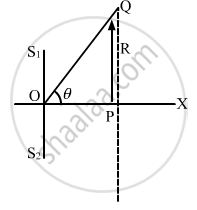
Let the man travel a distance of \[\left( y \right)\]parallel to the y-axis and let \[\left( d \right)\] be the distance between the two speakers. The man is standing at a distance of \[\left( D \right)\]from the origin.
The path difference (x) between the two sound waves reaching the man is given by :
\[x = S_2 Q - S_1 Q = \frac{yd}{D}\]
Angle made by man with the origin :
\[\theta = \frac{y}{D}\]
Given:
d = 2 m
(a) For minimum intensity:
The destructive interference of sound (minimum intensity) takes place if the path difference is an odd integral
multiple of half of the wavelength.
\[\therefore x = (2n + 1)\left( \frac{\lambda}{2} \right)\]
\[\text { For } \left( n = 0 \right)\]
\[ \therefore \frac{yd}{D} = \frac{\lambda}{2}\]
\[\left( \because \theta = \frac{y}{D} \right)\]
\[ \therefore \theta d = \frac{\lambda}{2}\]
\[ \therefore \theta = \frac{\lambda}{2d} = \frac{0 . 55}{4} = 0 . 1375 \text{ rad }\]
\[ \Rightarrow \theta = 0 . 1375 \times (57 . 1)^\circ= 7 . 9^\circ\]
(b) For maximum intensity:
The constructive interference of sound (maximum intensity) takes place if the path difference is an integral multiple of the wavelength.
\[x = n\lambda\]
\[\text { For } \left( n = 1 \right): \]
\[ \Rightarrow \frac{yd}{D} = \lambda\]
\[ \Rightarrow \theta = \frac{\lambda}{d}\]
\[ \Rightarrow \theta = \frac{0 . 55}{2} = 0 . 275 \text { rad }\]
\[ \therefore \theta = 16^\circ\]
(c) The more number of maxima is given by the path difference :
\[\frac{yd}{D} = 2\lambda, 3\lambda, 4\lambda, . . . . . \]
\[ \Rightarrow \frac{y}{D} = \theta = 32^\circ, 64^\circ, 128^\circ\]
He will hear two more maxima at 32° and 64° because the maximum value of θ may be 90°.
APPEARS IN
संबंधित प्रश्न
When we clap our hands, the sound produced is best described by Here p denotes the change in pressure from the equilibrium value.
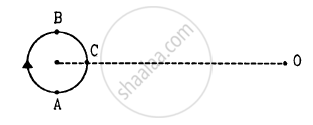
A small source of sounds moves on a circle as shown in figure and an observer is sitting at O. Let \[v_1, v_2, v_3\] be the frequencies heard when the source is at A, B and C respectively.
A listener is at rest with respect to the source of sound. A wind starts blowing along the line joining the source and the observer. Which of the following quantities do not change?
(a) Frequency
(b) Velocity of sound
(c) Wavelength
(d) Time period
A person can hear sound waves in the frequency range 20 Hz to 20 kHz. Find the minimum and the maximum wavelengths of sound that is audible to the person. The speed of sound is 360 m s−1.
Find the minimum and maximum wavelengths of sound in water that is in the audible range (20−20000 Hz) for an average human ear. Speed of sound in water = 1450 m s−1.
Calculate the bulk modulus of air from the following data about a sound wave of wavelength 35 cm travelling in air. The pressure at a point varies between (1.0 × 105 ± 14) Pa and the particles of the air vibrate in simple harmonic motion of amplitude 5.5 × 10−6 m.
The noise level in a classroom in absence of the teacher is 50 dB when 50 students are present. Assuming that on the average each student output same sound energy per second, what will be the noise level if the number of students is increased to 100?
A source of sound S and detector D are placed at some distance from one another. a big cardboard is placed near hte detector and perpendicular to the line SD as shown in figure. It is gradually moved away and it is found that the intensity changes from a maximum to a minimum as the board is moved through a distance of 20 cm. Find the frequency of the sound emitted. Velocity of sound in air is 336 m s−1.
Two coherent narrow slits emitting sound of wavelength λ in the same phase are placed parallel to each other at a small separation of 2λ. The sound is detected by moving a detector on the screen ∑ at a distance D(>>λ) from the slit S1 as shown in figure. Find the distance x such that the intensity at P is equal to the intensity at O.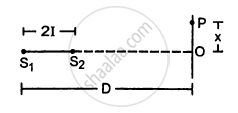
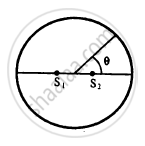
Figure shown two coherent sources S1 and S2 which emit sound of wavelength λ in phase. The separation between the sources is 3λ. A circular wire of large radius is placed in such way that S1,S2 is at the centre of the wire. Find the angular positions θ on the wire for which constructive interference takes place.
In a standing wave pattern in a vibrating air column, nodes are formed at a distance of 4.0 cm. If the speed of sound in air is 328 m s−1, what is the frequency of the source?
A train running at 108 km h−1 towards east whistles at a dominant frequency of 500 Hz. Speed of sound in air is 340 m/s. What frequency will a passenger sitting near the open window hear? (b) What frequency will a person standing near the track hear whom the train has just passed? (c) A wind starts blowing towards east at a speed of 36 km h−1. Calculate the frequencies heard by the passenger in the train and by the person standing near the track.
A person standing on a road sends a sound signal to the driver of a car going away from him at a speed of 72 km h−1. The signal travelling at 330 m s−1 in air and having a frequency of 1600 Hz gets reflected from the body of the car and returns. Find the frequency of the reflected signal as heard by the person.
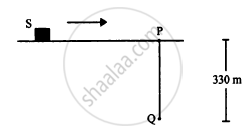
Figure shows a source of sound moving along X-axis at a speed of 22 m s−1continuously emitting a sound of frequency 2.0 kHz which travels in air at a speed of 330 m s−1. A listener Q stands on the Y-axis at a distance of 330 m from the origin. At t = 0, the sources crosses the origin P. (a) When does the sound emitted from the source at P reach the listener Q? (b) What will be the frequency heard by the listener at this instant? (c) Where will the source be at this instant?
A small source of sound S of frequency 500 Hz is attached to the end of a light string and is whirled in a vertical circle of radius 1.6 m. The string just remains tight when the source is at the highest point. (a) An observer is located in the same vertical plane at a large distance and at the same height as the centre of the circle. The speed of sound in air = 330 m s−1 and g = 10 m s−2. Find the maximum frequency heard by the observer. (b) An observer is situated at a large distance vertically above the centre of the circle. Find the frequency heard by the observer corresponding to the sound emitted by the source when it is at the same height as the centre.

For the propagation of longitudinal waves, the medium must have
- elasticity
- mass
- inertia
- force of cohesion
Equation of a plane progressive wave is given by `y = 0.6 sin 2π (t - x/2)`. On reflection from a denser medium its amplitude becomes 2/3 of the amplitude of the incident wave. The equation of the reflected wave is ______.
In the wave equation
`y = 0.5sin (2pi)/lambda(400t - x)m`
the velocity of the wave will be ______.
