Advertisements
Advertisements
प्रश्न
Using integration, find the area of the region {(x, y) : x2 + y2 ≤ 1 ≤ x + y}.
उत्तर
x2 + y2 = 1 represents a circle with centre at (0, 0) and radius as 1 unit.
Since, x2 + y2 ≤ 1, so the region represents the interior of the circle x2 + y2 = 1.
x + y = 1 is the equation of a straight line cutting X and Y axes at (1, 0) and (0, 1) respectively.
So, the shaded region is as follows:

\[\therefore \text { Area of the shaded region } = \int_0^1 \sqrt{1 - x^2}dx - \int_0^1 \left( 1 - x \right)dx\]
\[ = \left[ \frac{x}{2}\sqrt{1 - x^2} + \frac{1}{2} \sin^{- 1} \left( \frac{x}{1} \right) - x + \frac{x^2}{2} \right]_0^1 \]
\[ = \left[ \frac{1}{2}\sqrt{1 - 1} + \frac{1}{2} \sin^{- 1} \left( \frac{1}{1} \right) - 1 + \frac{1}{2} \right] - \left[ \frac{0}{2}\sqrt{1 - 0} + \frac{1}{2} \sin^{- 1} \left( \frac{0}{1} \right) - 0 + \frac{0}{2} \right]\]
\[ = \frac{\pi}{4} - 1 + \frac{1}{2}\]
\[ = \frac{\pi - 4 + 2}{4}\]
\[ = \frac{\pi - 2}{4}\text { units }\]
APPEARS IN
संबंधित प्रश्न
Find the area of the region bounded by y2 = 9x, x = 2, x = 4 and the x-axis in the first quadrant.
Area lying in the first quadrant and bounded by the circle x2 + y2 = 4 and the lines x = 0 and x = 2 is ______.
Find the area under the given curve and given line:
y = x2, x = 1, x = 2 and x-axis
Find the equation of an ellipse whose latus rectum is 8 and eccentricity is `1/3`
Draw a rough sketch and find the area bounded by the curve x2 = y and x + y = 2.
Find the area of the region bounded by the following curves, the X-axis and the given lines: 2y = 5x + 7, x = 2, x = 8
The area of the region bounded by y2 = 4x, the X-axis and the lines x = 1 and x = 4 is _______.
Using definite integration, area of the circle x2 + y2 = 49 is _______.
Fill in the blank :
The area of the region bounded by the curve x2 = y, the X-axis and the lines x = 3 and x = 9 is _______.
If the curve, under consideration, is below the X-axis, then the area bounded by curve, X-axis and lines x = a, x = b is positive.
State whether the following is True or False :
The area of the portion lying above the X-axis is positive.
Solve the following :
Find the area of the region bounded by the curve xy = c2, the X-axis, and the lines x = c, x = 2c.
Solve the following :
Find the area of the region bounded by y = x2, the X-axis and x = 1, x = 4.
Choose the correct alternative:
Area of the region bounded by the curve x2 = 8y, the positive Y-axis lying in the first quadrant and the lines y = 4 and y = 9 is ______
Choose the correct alternative:
Area of the region bounded by x = y4, y = 1 and y = 5 and the Y-axis lying in the first quadrant is ______
The area of the shaded region bounded by two curves y = f(x), and y = g(x) and X-axis is `int_"a"^"b" "f"(x) "d"x + int_"a"^"b" "g"(x) "d"x`
The area bounded by the parabola x2 = 9y and the lines y = 4 and y = 9 in the first quadrant is ______
If `int_0^(pi/2) log (cos x) "dx" = - pi/2 log 2,` then `int_0^(pi/2) log (cosec x)`dx = ?
The area of the region bounded by the curve y = 4x3 − 6x2 + 4x + 1 and the lines x = 1, x = 5 and X-axis is ____________.
The area bounded by y = `27/x^3`, X-axis and the ordinates x = 1, x = 3 is ______
`int "e"^x ((sqrt(1 - x^2) * sin^-1 x + 1)/sqrt(1 - x^2))`dx = ________.
If a2 + b2 + c2 = – 2 and f(x) = `|(1 + a^2x, (1 + b^2)x, (1 + c^2)x),((1 + a^2)x, 1 + b^2x, (1 + c^2)x),((1 + a^2)x, (1 + b^2)x, 1 + c^2x)|` then f(x) is a polynomial of degree
The area bounded by the x-axis and the curve y = 4x – x2 – 3 is ______.
Area bounded by y = sec2x, x = `π/6`, x = `π/3` and x-axis is ______.
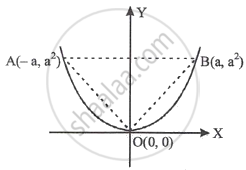
The figure shows as triangle AOB and the parabola y = x2. The ratio of the area of the triangle AOB to the area of the region AOB of the parabola y = x2 is equal to ______.
The area (in sq. units) of the region {(x, y) : y2 ≥ 2x and x2 + y2 ≤ 4x, x ≥ 0, y ≥ 0} is ______.
The area bounded by the curve | x | + y = 1 and X-axis is ______.
Find the area of the region lying in the first quadrant and bounded by y = 4x2, x = 0,y = 2 and y = 4.
