Advertisements
Advertisements
प्रश्न
Draw a rough sketch and find the area bounded by the curve x2 = y and x + y = 2.
उत्तर
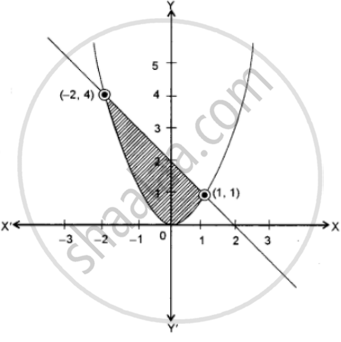
The given curves are: x2 = y
Which is an upward parabola with vertex at origin
And line x + y = 2 ⇒ y = 2 – x
x2 = 2 – x
⇒ x2 + x – 2 = 0
⇒ (x + 2)(x – 1) = 0
⇒ x = -2 and x = 1
Now, y = 2-(-2) = 4
and y = 2 – 1 ⇒ y = 1
⇒ y = 4 and y = 1
Thus, the points of intersection are (-2, 4) and (1, 1)
The required area of the shaded region
`= int_-2^1 (2 - "x") "dx" - int_-2^1 "x"^2 "dx"`
`= |2"x" - "x"^2/2|_-2^1 - |"x"^3/3|_-2^1`
`= 2 - 1/2 + 4 + 4/2 - 1/3 - 8/3`
`= (12 - 3 + 24 + 12 - 2 - 16)/6`
`= 9/2` sq.units
APPEARS IN
संबंधित प्रश्न
Find the area of the region in the first quadrant enclosed by the x-axis, the line y = x and the circle x2 + y2 = 32.
Find the area of the region bounded by the curve y2 = x and the lines x = 1, x = 4 and the x-axis.
Find the area of the region bounded by y2 = 9x, x = 2, x = 4 and the x-axis in the first quadrant.
Find the area of the region bounded by x2 = 4y, y = 2, y = 4 and the y-axis in the first quadrant.
Find the area under the given curve and given line:
y = x4, x = 1, x = 5 and x-axis
Find the area enclosed between the parabola y2 = 4ax and the line y = mx
Using the method of integration, find the area of the triangle ABC, coordinates of whose vertices are A (4 , 1), B (6, 6) and C (8, 4).
Find the area bounded by the circle x2 + y2 = 16 and the line `sqrt3 y = x` in the first quadrant, using integration.
The area of the region bounded by y2 = 4x, the X-axis and the lines x = 1 and x = 4 is _______.
If the curve, under consideration, is below the X-axis, then the area bounded by curve, X-axis and lines x = a, x = b is positive.
Area of the region bounded by the curve x = y2, the positive Y axis and the lines y = 1 and y = 3 is ______
Choose the correct alternative:
Area of the region bounded by the curve x2 = 8y, the positive Y-axis lying in the first quadrant and the lines y = 4 and y = 9 is ______
State whether the following statement is True or False:
The equation of the area of the circle is `x^2/"a"^2 + y^2/"b"^2` = 1
The area bounded by the parabola x2 = 9y and the lines y = 4 and y = 9 in the first quadrant is ______
The area of the region bounded by the curve y2 = 4x, the X axis and the lines x = 1 and x = 4 is ______
The area of the region x2 = 4y, y = 1 and y = 2 and the Y axis lying in the first quadrant is ______
Find area of the region bounded by 2x + 4y = 10, y = 2 and y = 4 and the Y-axis lying in the first quadrant
Find the area of the circle x2 + y2 = 62
The area bounded by y = `27/x^3`, X-axis and the ordinates x = 1, x = 3 is ______
Which equation below represents a parabola that opens upward with a vertex at (0, – 5)?
The equation of curve through the point (1, 0), if the slope of the tangent to t e curve at any point (x, y) is `(y - 1)/(x^2 + x)`, is
If a2 + b2 + c2 = – 2 and f(x) = `|(1 + a^2x, (1 + b^2)x, (1 + c^2)x),((1 + a^2)x, 1 + b^2x, (1 + c^2)x),((1 + a^2)x, (1 + b^2)x, 1 + c^2x)|` then f(x) is a polynomial of degree
The area of the circle `x^2 + y^2 = 16`, exterior to the parabola `y = 6x`
The area of the region bounded by the curve y = sin x and the x-axis in [–π, π] is ______.
If area of the region bounded by y ≥ cot( cot–1|In|e|x|) and x2 + y2 – 6 |x| – 6|y| + 9 ≤ 0, is λπ, then λ is ______.
Area bounded by y = sec2x, x = `π/6`, x = `π/3` and x-axis is ______.
Find the area of the region lying in the first quadrant and bounded by y = 4x2, x = 0,y = 2 and y = 4.
