Advertisements
Advertisements
प्रश्न
Verify that points P(–2, 2), Q(2, 2) and R(2, 7) are vertices of a right angled triangle.
उत्तर
Distance between two points = `sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2)`
By distance formula,
`"PQ" = sqrt([2 - (-2)]^2 + (2 - 2)^2)`
`"PQ" = sqrt((2 + 2)^2 + (0)^2)`
`"PQ" = sqrt((4)^2)`
PQ = 4 ...(i)
`"QR" = sqrt((2 - 2)^2 + (7 - 2)^2)`
`"QR" = sqrt((0)^2 + (5)^2)`
`"QR" = sqrt((5)^2)`
QR = 5 ...(ii)
`"PR" = sqrt([2 - (-2)]^2 + (7 - 2)^2)`
`"PR" = sqrt((2 + 2)^2 + (5)^2)`
`"PR" = sqrt((4)^2 + (5)^2)`
`"PR" = sqrt(16 + 25)`
`"PR" = sqrt(41)`
Now, PR2 = `(sqrt(41))^2` = 41 ...(iii)
From (i) and (ii),
∴ PQ2 + QR2 = 42 + 52 = 16 + 25 = 41
∴ PR2 = PQ2 + QR2 ...[From (iii)]
∴ ΔPQR is a right angled triangle. ...[Converse of Pythagoras theorem]
∴ Point P, Q, and R are the vertices of a right angled triangle.
APPEARS IN
संबंधित प्रश्न
A (5, 4), B (-3, -2) and C (1, -8) are the vertices of a triangle ABC. Find the equations of the median AD and line parallel to AC passing through the point B.
Find the slope and y-intercept of the line:
ax – by = 0
Is the line 3x + 4y + 7 = 0 perpendicular to the line 28x – 21y + 50 = 0?
Is the line x – 3y = 4 perpendicular to the line 3x – y = 7?
- Write down the equation of the line AB, through (3, 2) and perpendicular to the line 2y = 3x + 5.
- AB meets the x-axis at A and the y-axis at B. Write down the co-ordinates of A and B. Calculate the area of triangle OAB, where O is the origin.
The line 4x − 3y + 12 = 0 meets x-axis at A. Write the co-ordinates of A. Determine the equation of the line through A and perpendicular to 4x – 3y + 12 = 0.
The point P is the foot of perpendicular from A(−5, 7) to the line whose equation is 2x – 3y + 18 = 0. Determine :
- the equation of the line AP.
- the co-ordinates of P.
The points A, B and C are (4, 0), (2, 2) and (0, 6) respectively. Find the equations of AB and BC. If AB cuts the y-axis at P and BC cuts the x-axis at Q, find the co-ordinates of P and Q.
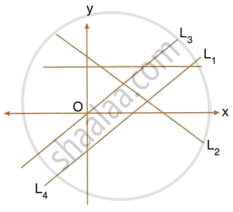
Match the equations A, B, C and D with the lines L1, L2, L3 and L4, whose graphs are roughly drawn in the given diagram.
A ≡ y = 2x;
B ≡ y – 2x + 2 = 0;
C ≡ 3x + 2y = 6;
D ≡ y = 2
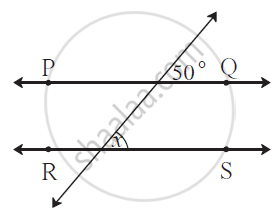
In the figure, line PQ || line RS. Using the information given
in the figure find the value of x.
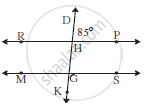
In the adjoining figure line RP ||line MS , line DK is a transversal . If ∠DHP = 85° find ∠RHG and ∠HGS.
Line PQ is parallel to line RS where points P,Q,R and S have
co-ordinates (2, 4), (3, 6), (3, 1) and (5, k) respectively. Find value of k.
Find the equation of the line that has x-intercept = –3 and is perpendicular to 3x + 5y = 1.
Three vertices of a parallelogram ABCD taken in order are A(3, 6), B(5, 10) and C(3, 2), find:
- the co-ordinates of the fourth vertex D.
- length of diagonal BD.
- equation of side AB of the parallelogram ABCD.
In the figure, given, ABC is a triangle and BC is parallel to the y-axis. AB and AC intersect the y-axis at P and Q respectively.

- Write the co-ordinates of A.
- Find the length of AB and AC.
- Find the radio in which Q divides AC.
- Find the equation of the line AC.
If (4,-3) is a point on line 5x +8y = c, find the value of c.
