Advertisements
Advertisements
प्रश्न
Which of the following equation represents a line equally inclined to the axes?
विकल्प
2x – 3y + 7 = 0
x – y = 7
x = 7
y = –7
उत्तर
x – y = 7
Explanation:
A line equally inclined to the x-axis and y-axis means that the line makes an angle of 45° or 135° with the positive x-axis. Such lines have slopes of 1 or –1.
For the line x – y = 7:
- Rearrange to slope-intercept form: y = x – 7
- The slope is 1, indicating the line is inclined at 45° to the positive x-axis, hence equally inclined to both axes.
APPEARS IN
संबंधित प्रश्न
State, true or false :
If the point (2, a) lies on the line 2x – y = 3, then a = 5.
The line y = 3x – 2 bisects the join of (a, 3) and (2, −5), find the value of a.
The line y = mx + 8 contains the point (−4, 4), calculate the value of m.
Find the equation of the line, whose x-intercept = −4 and y-intercept = 6
A line 5x + 3y + 15 = 0 meets y-axis at point P. Find the co-ordinates of points P. Find the equation of a line through P and perpendicular to x – 3y + 4 = 0.
A straight line passes through the point (3, 2) and the portion of this line, intercepted between the positive axes, is bisected at this point. Find the equation of the line.
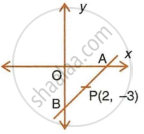
A and B are two points on the x-axis and y-axis respectively. P(2, −3) is the mid point of AB. Find the
- co-ordinates of A and B
- slope of line AB
- equation of line AB.
The line y = 6- `(3"x")/2` passes through the point (r,3). Find the value of r.
Find the value of a line parallel to the following line:
`"x"/4 +"y"/3` = 1
Find the value of ‘a’ for which the following points A (a, 3), B (2, 1) and C (5, a) are collinear. Hence find the equation of the line.
