HSC Science (General)
HSC Arts (English Medium)
HSC Science (Electronics)
HSC Science (Computer Science)
HSC Arts (Marathi Medium)
Academic Year: 2022-2023
Date & Time: 3rd March 2023, 11:00 am
Duration: 3h
Advertisements
General instructions:
The question paper is divided into FOUR sections.
- Section A:
Q.1 contains Eight multiple-choice type questions, each carrying Two marks.
Q.2 contains Four very short answer type questions, each carrying one mark. - Section B: Q.3 to Q. 14 contain Twelve short answer type questions, each carrying Two marks. (Attempt any Eight)
- Section C: Q.15 to Q. 26 contain Twelve short answer type questions, each carrying Three marks. (Attempt any Eight)
- Section D: Q.27 to Q. 34 contain Eight long answer type questions, each carrying Four marks. (Attempt any Five)
- Use of log table is allowed. Use of calculator is not allowed.
- Figures to the right indicate full marks.
- Use of graph paper is not necessary. Only rough sketch of graph is expected.
- For each multiple-choice type of question, it is mandatory to write the correct answer along with its alphabet, e.g. (a).../(b).../(c).../(d)..., etc. No marks shall be given if ONLY the correct answer or the alphabet of correct answer is written. Only the first attempt will be considered for evaluation.
- Start answer to each section on a new page.
If p ∧ q is F, p → q is F then the truth values of p and q are ________.
T, T
T, F
F, T
F, F
Chapter: [0.01] Mathematical Logic [0.011000000000000001] Mathematical Logic
Select the correct option from the given alternatives:
In ΔABC if c2 + a2 – b2 = ac, then ∠B = ____.
`π/4`
`π/3`
`π/2`
`π/6`
Chapter: [0.013000000000000001] Trigonometric Functions
The area of the triangle with vertices (1, 2, 0), (1, 0, 2) and (0, 3, 1) in sq. unit is ______.
`sqrt(5)`
`sqrt(7)`
`sqrt(6)`
`sqrt(3)`
Chapter: [0.015] Vectors
If the corner points of the feasible solution are (0, 10), (2, 2) and (4, 0), then the point of minimum z = 3x + 2y is ______.
(2, 2)
(2, 2)
(0, 10)
(0, 10)
(4, 0)
(4, 0)
(3, 4)
(2, 4)
Chapter: [0.017] Linear Programming
If y is a function of x and log (x + y) = 2xy, then the value of y'(0) = ______.
2
0
–1
1
Chapter: [0.021] Differentiation
`int cos^3x dx` = ______.
`1/12 sin 3x + 3/4 sin x + c`
`1/12 sin 3x + 1/4 sin x + c`
`1/12 sin 3x - 3/4 sin x + c`
`1/12 sin 3x - 1/4 sin x + c`
Chapter: [0.023] Indefinite Integration [0.15] Integration
The solution of the differential equation `dx/dt = (xlogx)/t` is ______.
x = ect
x + ect = 0
x = et + t
xect = 0
Chapter: [0.026000000000000002] Differential Equations
Let the probability mass function (p.m.f.) of a random variable X be P(X = x) = `""^4C_x (5/9)^x xx (4/9)^(4 - x)`, for x = 0, 1, 2, 3, 4 then E(X) is equal to ______.
`20/9`
`9/20`
`12/9`
`9/25`
Chapter: [0.027999999999999997] Binomial Distribution [0.2] Bernoulli Trials and Binomial Distribution
Write the joint equation of co-ordinate axes.
Chapter: [0.013999999999999999] Pair of Straight Lines
Find the values of c which satisfy `|"c"overline"u"|` = 3 where `overline"u" = hat"i" + 2hat"j" + 3hat"k"`.
Chapter: [0.015] Vectors
Write `int cotx dx`.
Chapter: [0.023] Indefinite Integration [0.15] Integration
State the degree of differential equation `"e"^((dy)/(dx)) + (dy)/(dx)` = x
Chapter: [0.026000000000000002] Differential Equations [0.17] Differential Equation
Write converse, inverse and contrapositive of the following statement.
If x < y then x2 < y2 (x, y ∈ R)
Chapter: [0.011000000000000001] Mathematical Logic
Advertisements
If A = `[("x",0,0),(0,"y",0),(0,0,"z")]` is a non-singular matrix, then find A−1 by using elementary row transformations. Hence, find the inverse of `[(2,0,0),(0,1,0),(0,0,-1)]`
Chapter: [0.012] Matrics
Find the Cartesian co-ordinates of the point whose polar co-ordinates are:
`(sqrt(2), pi/4)`
Chapter: [0.013000000000000001] Trigonometric Functions [0.03] Trigonometric Functions
If ax2 + 2hxy + by2 = 0 represents a pair of lines and h2 = ab ≠ 0 then find the ratio of their slopes.
Chapter: [0.013999999999999999] Pair of Straight Lines
If `overlinea, overlineb, overlinec` are the position vectors of the points A, B, C respectively and `5overlinea + 3overlineb - 8overlinec = overline0` then find the ratio in which the point C divides the line segment AB.
Chapter: [0.015] Vectors [0.07] Vectors
Find the feasible solution of the following inequation:
2x + 3y ≤ 6, x + y ≥ 2, x ≥ 0, y ≥ 0
Chapter: [0.017] Linear Programming
Show that the function f(x) = x3 + 10x + 7 for x ∈ R is strictly increasing
Chapter: [0.022000000000000002] Applications of Derivatives [0.14] Applications of Derivative
Evaluate: `int_0^(pi/2) sqrt(1 - cos 4x) "d"x`
Chapter: [0.024] Definite Integration
Find the area of the region bounded by the curve y2 = 4x, the X-axis and the lines x = 1, x = 4 for y ≥ 0.
Chapter: [0.025] Application of Definite Integration
Solve the following differential equation:
cos x . cos y dy − sin x . sin y dx = 0
Chapter: [0.026000000000000002] Differential Equations
Find the mean of number randomly selected from 1 to 15.
Chapter: [0.027000000000000003] Probability Distributions [0.19] Probability Distribution
Find the area of the region bounded by the curve y = x2 and the line y = 4.
Chapter: [0.025] Application of Definite Integration
Find the general solution of sin θ + sin 3θ + sin 5θ = 0
Chapter: [0.013000000000000001] Trigonometric Functions
If –1 ≤ x ≤ 1, the prove that sin–1 x + cos–1 x = `π/2`
Chapter: [0.013000000000000001] Trigonometric Functions [0.03] Trigonometric Functions
If θ is the acute angle between the lines represented by ax2 + 2hxy + by2 = 0 then prove that tan θ = `|(2sqrt(h^2 - ab))/(a + b)|`
Chapter: [0.013999999999999999] Pair of Straight Lines
Find the direction ratios of a vector perpendicular to the two lines whose direction ratios are - 2, 1, - 1 and - 3, - 4, 1
Chapter: [0.015] Vectors
Advertisements
Find the shortest distance between the lines `(x-1)/2=(y-2)/3=(z-3)/4 and (x-2)/3=(y-4)/4=(z-5)/5`
Chapter: [0.04] Pair of Straight Lines
Lines `overliner = (hati + hatj - hatk) + λ(2hati - 2hatj + hatk)` and `overliner = (4hati - 3hatj + 2hatk) + μ(hati - 2hatj + 2hatk)` are coplanar. Find the equation of the plane determined by them.
Chapter: [0.016] Line and Plane [0.1] Plane
If y = `sqrt(tan x + sqrt(tanx + sqrt(tanx + .... + ∞)`, then show that `dy/dx = (sec^2x)/(2y - 1)`.
Find `dy/dx` at x = 0.
Chapter: [0.021] Differentiation [0.13] Differentiation
Find the approximate value of sin (30° 30′). Give that 1° = 0.0175c and cos 30° = 0.866
Chapter: [0.022000000000000002] Applications of Derivatives [0.14] Applications of Derivative
Evaluate the following : `int x tan^-1 x .dx`
Chapter: [0.023] Indefinite Integration [0.15] Integration
Find the particular solution of the differential equation `dy/dx` = e2y cos x, when x = `π/6`, y = 0
Chapter: [0.026000000000000002] Differential Equations
For the following probability density function of a random variable X, find P(X < 1).
`{:(f(x) = (x + 2)/18,";" "for" -2 < x < 4),( = 0,"," "otherwise"):}`
Chapter: [0.027000000000000003] Probability Distributions
For the following probability density function of a random variable X, find P(|X| < 1).
`{:(f(x) = (x + 2)/18,";" "for" -2 < x < 4),( = 0,"," "otherwise"):}`
Chapter: [0.027000000000000003] Probability Distributions
A die is thrown 6 times. If ‘getting an odd number’ is a success, find the probability of at least 5 successes.
Chapter: [0.027999999999999997] Binomial Distribution
Simplify the given circuit by writing its logical expression. Also, write your conclusion.
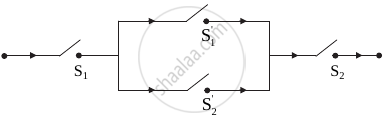
Chapter: [0.01] Mathematical Logic [0.011000000000000001] Mathematical Logic
If A = `[(1, 2),(3, 4)]` verify that A (adj A) = (adj A) A = |A| I
Chapter: [0.012] Matrics
Prove that the volume of a tetrahedron with coterminus edges `overlinea, overlineb` and `overlinec` is `1/6[(overlinea, overlineb, overlinec)]`.
Hence, find the volume of tetrahedron whose coterminus edges are `overlinea = hati + 2hatj + 3hatk, overlineb = -hati + hatj + 2hatk` and `overlinec = 2hati + hatj + 4hatk`.
Chapter: [0.015] Vectors [0.07] Vectors
Find the length of the perpendicular drawn from the point P(3, 2, 1) to the line `overliner = (7hati + 7hatj + 6hatk) + λ(-2hati + 2hatj + 3hatk)`
Chapter: [0.016] Line and Plane [0.09] Line
If y = cos(m cos–1x), then show that `(1 - x^2) ("d"^2y)/("d"x^2) - x("d"y)/("d"x) + "m"^2y` = 0
Chapter: [0.021] Differentiation
Verify Lagrange’s mean value theorem for the function f(x) = `sqrt(x + 4)` on the interval [0, 5].
Chapter: [0.022000000000000002] Applications of Derivatives
Evaluate: `int (2x^2 - 3)/((x^2 - 5)(x^2 + 4))dx`
Chapter: [0.023] Indefinite Integration [0.15] Integration
Prove that: `int_0^(2a) f(x)dx = int_0^a f(x)dx + int_0^a f(2a - x)dx`
Chapter: [0.024] Definite Integration
Other Solutions
Submit Question Paper
Help us maintain new question papers on Shaalaa.com, so we can continue to help studentsonly jpg, png and pdf files
Maharashtra State Board previous year question papers 12th Standard Board Exam Mathematics and Statistics with solutions 2022 - 2023
Previous year Question paper for Maharashtra State Board 12th Standard Board Exam Maths and Stats-2023 is solved by experts. Solved question papers gives you the chance to check yourself after your mock test.
By referring the question paper Solutions for Mathematics and Statistics, you can scale your preparation level and work on your weak areas. It will also help the candidates in developing the time-management skills. Practice makes perfect, and there is no better way to practice than to attempt previous year question paper solutions of Maharashtra State Board 12th Standard Board Exam.
How Maharashtra State Board 12th Standard Board Exam Question Paper solutions Help Students ?
• Question paper solutions for Mathematics and Statistics will helps students to prepare for exam.
• Question paper with answer will boost students confidence in exam time and also give you an idea About the important questions and topics to be prepared for the board exam.
• For finding solution of question papers no need to refer so multiple sources like textbook or guides.
