Advertisements
Advertisements
प्रश्न
A field is in the shape of a quadrilateral ABCD in which side AB = 18 m, side AD = 24 m, side BC = 40m, DC = 50 m and angle A = 90°. Find the area of the field.
उत्तर
Since ∠A = 90°
By Pythagorus Theorem,
In ∆ABD,
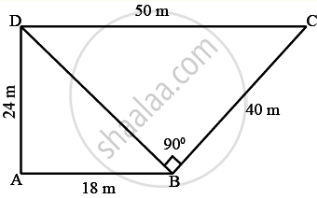
Now, the area of ΔABD = `1/2 (18)(24)`
= (18) (12) = 216 m2
In ΔABD
BD = `sqrt("AB"^2 + "AD"^2) = sqrt(18^2 + 24^2)`
= `sqrt(324 + 576) = sqrt(900) = 30`m.
S = `120/20 = 60 cm^2`
=`sqrt( 60xx10xx20xx30)`
= `sqrt(10xx2xx3xx10xx10xx2xx10xx3)`
= 10 × 10 × 6
= 600 cm2
Area of quadrilateral ABCD
= 816cm2
APPEARS IN
संबंधित प्रश्न
Calculate the area and the height of an equilateral triangle whose perimeter is 60 cm.
The length of the sides of a triangle are in the ratio 3: 4: 5. Find the area of the triangle if its perimeter is 144 cm.
The area of an equilateral triangle is 36`sqrt3` sq. cm. Find its perimeter.
The perimeter of a triangle is 450 m and its side are in the ratio 12: 5: 13. Find the area of the triangle.
Two sides of a triangle are 6.4 m and 4.8 m. If the height of the triangle corresponding to 4.8 m side is 6 m;
find :
(i) area of the triangle ;
(ii) height of the triangle corresponding to 6.4 m sides.
The table given below contains some measures of the triangle. Find the unknown values.
| Side 1 | Side 2 | Side 3 | Perimeter |
| ? | 8 m | 3 m | 17 m |
Find the perimeter of A scalene triangle with sides 7 m, 8 m, 10 m
Find the perimeter of An equilateral triangle with side 6 cm
A piece of wire is 36 cm long. What will be the length of each side if we form an equilateral triangle
The perimeter of a triangle is 28 cm. One of it’s sides is 8cm. Write all the sides of the possible isosceles triangles with these measurements.
