Advertisements
Advertisements
प्रश्न
A field is in the shape of a trapezium having parallel sides 90 m and 30 m. These sides meet the third side at right angles. The length of the fourth side is 100 m. If it costs Rs 4 to plough 1 m2 of the field, find the total cost of ploughing the field.
उत्तर
Given: In the trapezium ABCD, the two parallel sides are AB = 90 m, CD = 30 m and EC ⊥ AB.
So, EB = AB – EA = 90 m – 30 m = 60 m
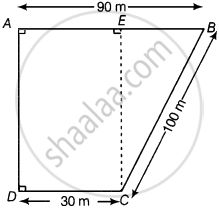
Now, in triangle BEC,
(BC)2 = (BE)2 + (EC)2
1002 = 602 + (EC)2
(EC)2 = 10000 – 3600
(EC)2 = 6400
EC = `sqrt(6400)`
EC = 80 m
Now, area of trapezium ABCD = `1/2` × (Sum of parallel sides) × (Distance between parallel sides)
= `1/2 xx (AB + CD) xx EC`
= `1/2 xx (90 + 30) xx 80`
= `1/2 xx 120 xx 80`
= 4800 m2
The cost of ploughing the field of 1 m2 is Rs. 4.
Now, The cost of ploughing the field of 4800 m2 area = 4800 × Rs. 4 = Rs. 19200.
Therefore, the total cost of plughing the field is Rs. 19200.
APPEARS IN
संबंधित प्रश्न
A traffic signal board, indicating ‘SCHOOL AHEAD’, is an equilateral triangle with side ‘a’. Find the area of the signal board, using Heron’s formula. If its perimeter is 180 cm, what will be the area of the signal board?
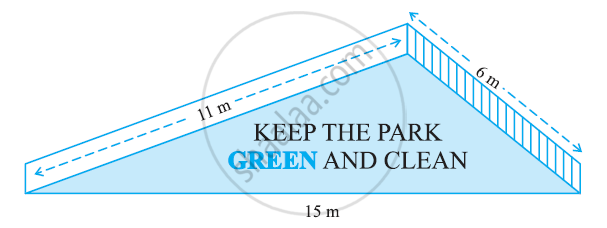
There is a slide in the park. One of its side walls has been painted in some colour with a message “KEEP THE PARK GREEN AND CLEAN” (see the given figure). If the sides of the wall are 15m, 11m, and 6m, find the area painted in colour.
A rhombus sheet, whose perimeter is 32 m and whose one diagonal is 10 m long, is painted on both sides at the rate of Rs 5 per m2. Find the cost of painting.
Find the area of a triangle whose sides are 3 cm, 4 cm and 5 cm respectively.
Determine whether the sets of points are collinear?
(a, b + c), (b, c + a) and (c, a + b)
The perimeter of a triangular plot is 600 m. If the sides are in the ratio 5 : 12 : 13, then find the area of the plot
If the side of a rhombus is 10 cm and one diagonal is 16 cm, the area of the rhombus is 96 cm2.
From a point in the interior of an equilateral triangle, perpendiculars are drawn on the three sides. The lengths of the perpendiculars are 14 cm, 10 cm and 6 cm. Find the area of the triangle.
How much paper of each shade is needed to make a kite given in the following figure, in which ABCD is a square with diagonal 44 cm.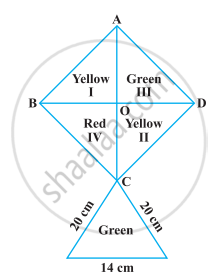
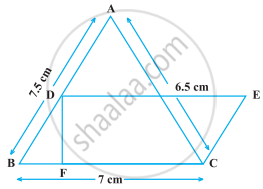
In the following figure, ∆ABC has sides AB = 7.5 cm, AC = 6.5 cm and BC = 7 cm. On base BC a parallelogram DBCE of same area as that of ∆ABC is constructed. Find the height DF of the parallelogram.