Advertisements
Advertisements
प्रश्न
In the following figure, ∆ABC has sides AB = 7.5 cm, AC = 6.5 cm and BC = 7 cm. On base BC a parallelogram DBCE of same area as that of ∆ABC is constructed. Find the height DF of the parallelogram.
Advertisements
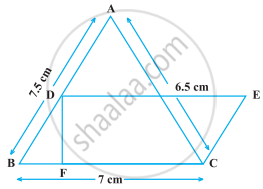
उत्तर
Now, first determine the area of ∆ABC.
The sides of a triangle are AB = a = 7.5 cm, BC = b = 7 cm and CA = c = 6.5 cm
Now, semi-perimeter of a triangle,
`s = (a + b + c)/2`
= `(7.5 + 7 + 6.5)/2`
= `21/2`
= 10.5 cm
∴ Area of ΔABC = `sqrt(s(s - a)(s - b)(s - c))` ...[By Heron’s formula]
= `sqrt(10.5(10.5 - 7.5)(10.5 - 7)(10.5 - 6.5))`
= `sqrt(10.5 xx 3 xx 3.5 xx 4)`
= `sqrt(441)`
= 21 cm2 ...(i)
Now, area of parallelogram BCED = Base × Height
= BC × DF
= 7 × DF ...(ii)
According to the question,
Area of ∆ABC = Area of parallelogram BCED
⇒ 21 = 7 × DF ...[From equations (i) and (ii)]
⇒ DF = `21/4` = 3 cm
Hence, the height of parallelogram is 3 cm.
APPEARS IN
संबंधित प्रश्न
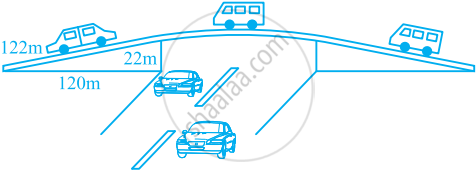
The triangular side walls of a flyover have been used for advertisements. The sides of the walls are 122m, 22m, and 120m (see the given figure). The advertisements yield an earning of ₹ 5000 per m2 per year. A company hired one of its walls for 3 months. How much rent did it pay?
An isosceles triangle has perimeter 30 cm and each of the equal sides is 12 cm. Find the area of the triangle.
The adjacent sides of a parallelogram ABCD measure 34 cm and 20 cm, and the diagonal AC measures 42 cm. Find the area of the parallelogram.
If the points A(– 3, 9), B(a, b) and C(4, – 5) are collinear and if a + b = 1, then find a and b
A triangular shaped glass with vertices at A(– 5, – 4), B(1, 6) and C(7, – 4) has to be painted. If one bucket of paint covers 6 square feet, how many buckets of paint will be required to paint the whole glass, if only one coat of paint is applied
The perimeter of a triangular plot is 600 m. If the sides are in the ratio 5 : 12 : 13, then find the area of the plot
An advertisement board is in the form of an isosceles triangle with perimeter 36 m and each of the equal sides are 13 m. Find the cost of painting it at ₹ 17.50 per square metre.
The semi-perimeter of a triangle having sides 15 cm, 20 cm and 25 cm is
The perimeter of an equilateral triangle is 30 cm. The area is
The perimeter of an isosceles triangle is 32 cm. The ratio of the equal side to its base is 3 : 2. Find the area of the triangle.
