Advertisements
Advertisements
प्रश्न
A force \[\vec{F} = \vec{v} \times \vec{A}\] is exerted on a particle in addition to the force of gravity, where \[\vec{v}\] is the velocity of the particle and \[\vec{A}\] is a constant vector in the horizontal direction. With what minimum speed, a particle of mass m be projected so that it continues to move without being defelected and with a constant velocity?
उत्तर
For the particle to move without being deflected and with constant velocity, the net force on the particle should be zero.
\[\vec{F} + m \vec{g} = 0\]
\[\Rightarrow \left( \vec{v} \times \vec{A} \right) + \vec{mg} = 0\]
\[ \Rightarrow \left( \vec{v} \times \vec{A} \right) = - \vec{mg}\]
\[\left| vA\sin\theta \right| = \left| mg \right|\]
\[\therefore v = \frac{mg}{A\sin\theta}\]
v will be minimum when sinθ = 1.
⇒ θ = 90°
\[\therefore v_{\text{min}} = \frac{mg}{A}\]
APPEARS IN
संबंधित प्रश्न
Two masses 8 kg and 12 kg are connected at the two ends of a light, inextensible string that goes over a frictionless pulley. Find the acceleration of the masses, and the tension in the string when the masses are released.
Explain why a cricketer moves his hands backwards while holding a catch.
A block of mass 15 kg is placed on a long trolley. The coefficient of static friction between the block and the trolley is 0.18. The trolley accelerates from rest with 0.5 ms–2 for 20 s and then moves with uniform velocity. Discuss the motion of the block as viewed by (a) a stationary observer on the ground, (b) an observer moving with the trolley.
A free 238U nucleus kept in a train emits an alpha particle. When the train is stationary, a nucleus decays and a passenger measures that the separation between the alpha particle and the recoiling nucleus becomes x at time t after the decay. If the decay takes place while the train is moving at a uniform velocity v, the distance between the alpha particle and the recoiling nucleus at a time t after the decay, as measured by the passenger, is
A block of mass 0.2 kg is suspended from the ceiling by a light string. A second block of mass 0.3 kg is suspended from the first block by another string. Find the tensions in the two strings. Take g = 10 m/s2.
Consider the situation shown in the following figure All the surfaces are frictionless and the string and the pulley are light. Find the magnitude of acceleration of the two blocks.

In the following figure, m1 = 5 kg, m2 = 2 kg and F = 1 N. Find the acceleration of either block. Describe the motion of m1 if the string breaks but F continues to act.
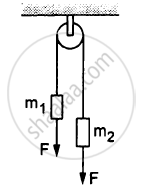
Consider the situation shown in the following figure. Both the pulleys and the string are light and all the surfaces are frictionless. (a) Find the acceleration of the mass M; (b) find the tension in the string; (c) calculate the force exerted by the clamp on the pulley A in the figure.
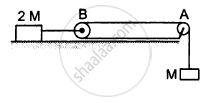
Find the mass M of the hanging block in the following figure that will prevent the smaller block from slipping over the triangular block. All the surfaces are frictionless and the strings and the pulleys are light.
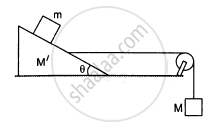
A block A can slide on a frictionless incline of angle θ and length l, kept inside an elevator going up with uniform velocity v in the following figure. Find the time taken by the block to slide down the length of the incline if it is released from the top of the incline.
Two bodies A and B of same mass are moving with velocities v and 2v, respectively. Compare their (i) inertia and (ii) momentum.
A motorcycle of mass 100 kg is running at 10 ms−1. If its engine develops an extra linear momentum of 2000 Ns, calculate the new velocity of a motorcycle.
Multiple Choice Question. Select the correct option.
The impulse of a body is equal to:
State Newton's second law of motion. Is Newton's first law of motion contained in Newton's second law of motion?
A stone is dropped from a cliff 98 m high.
How long will it take to fall to the foot of the cliff?
A stone is dropped from a cliff 98 m high.
What will be its speed when it strikes the ground?
A stone is thrown vertically upward with a velocity of 9.8 m/s. When will it reach the ground?
A body of mass 2 kg travels according to the law x(t) = pt + qt2 + rt3 where p = 3 ms−1, q = 4 ms−2 and r = 5 ms−3. The force acting on the body at t = 2 seconds is ______.
The motion of a particle of mass m is given by x = 0 for t < 0 s, x(t) = A sin 4 pt for 0 < t < (1/4) s (A > o), and x = 0 for t > (1/4) s. Which of the following statements is true?
- The force at t = (1/8) s on the particle is – 16π2 Am.
- The particle is acted upon by on impulse of magnitude 4π2 A m at t = 0 s and t = (1/4) s.
- The particle is not acted upon by any force.
- The particle is not acted upon by a constant force.
- There is no impulse acting on the particle.
