Advertisements
Advertisements
प्रश्न
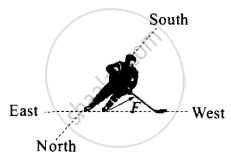
A hockey player is moving northward and suddenly turns westward with the same speed to avoid an opponent. The force that acts on the player is ______.
पर्याय
frictional force along westward.
muscle force along southward.
frictional force along south-west.
muscle force along south-west.
उत्तर
A hockey player is moving northward and suddenly turns westward with the same speed to avoid an opponent. The force that acts on the player is frictional force along south-west.
Explanation:
According to Newton’s second law of motion, only external forces can change the linear momentum of the system. The internal forces cannot change the linear momentum of system under consideration. If we take hockey players as a system, the external force which can change the direction of motion of the player is the force that must be friction between the ground and the shoes of player. The muscle force is the internal force, this cannot change the linear momentum of the player.
According to Newton’s Second Law, The rate of change of linear momentum of a body is equal to the external force applied on the body or F = dp/dt. So, the external force must be in the direction of change in momentum.
As shown in the diagram,

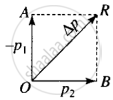
Let OA = p1
= Initial momentum of player northward
AB = p2
= Final momentum of the player towards west
Clearly OB = OA + AB

According to the problem, mass = 2 kg
The position of the particle is given here as a function of time, x(t) = pt + qt2 + rt3 By differentiating this equation w.r.t. time we get velocity of the particle as a function of time.
v = dx/dt = p + 2 qt + 3 rt2
If we again differentiate this equation w.r.t. time, we will get an acceleration of the particle as a function of time.
a = dv/dt = 0 + 2q + 6rt
At t = 2 s; a = 2q + 6 × 2 × r
= 2 q + 12 r
= 2 × 4 + 12 × 5
= 8 + 60
= 68 m/s
Force = F = ma
= 2 × 68
= 136 N
Change in momentum = p2 – p1
= AB – OA
= AB + (– OA)
= Clearly the change in momentum is OR will be along southwest, so the direction of force will also be along southwest.
APPEARS IN
संबंधित प्रश्न
A body of mass 0.40 kg moving initially with a constant speed of 10 m s–1 to the north is subject to a constant force of 8.0 N directed towards the south for 30 s. Take the instant the force is applied to be t = 0, the position of the body at that time to be x = 0, and predict its position at t = –5 s, 25 s, 100 s.
Two bodies of masses 10 kg and 20 kg respectively kept on a smooth, horizontal surface are tied to the ends of a light string. A horizontal force F = 600 N is applied to
- A,
- B along the direction of string. What is the tension in the string in each case?
A batsman deflects a ball by an angle of 45° without changing its initial speed which is equal to 54 km/h. What is the impulse imparted to the ball? (Mass of the ball is 0.15 kg.)
An aircraft executes a horizontal loop at a speed of 720 km/h with its wings banked at 15°. What is the radius of the loop?
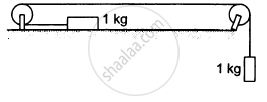
Calculate the tension in the string shown in the following figure. The pulley and the string are light and all the surfaces are frictionless. Take g = 10 m/s2.
A block is kept on the floor of an elevator at rest. The elevator starts descending with an acceleration of 12 m/s2. Find the displacement of the block during the first 0.2 s after the start. Take g = 10 m/s2.
How can Newton's first law of motion be obtained from the second law of motion?
Calculate the magnitude of force which when applied on a body of mass 0.5 kg produces an acceleration of 5 m s-2.
A pebble is thrown vertically upwards with a speed of 20 m s-1. How high will it be after 2 s? (Take g = 10 m s-2)
Prove mathematically F = ma
