Advertisements
Advertisements
प्रश्न
A man on the deck of a ship, 16m above water level, observe that that angle of elevation and depression respectively of the top and bottom of a cliff are 60° and 30° . Calculate the distance of the cliff from the ship and height of the cliff.
उत्तर
Let AB be the deck of the ship above the water level and DE be the cliff.
Now,
AB = 16msuch that CD = 16mand ∠BDA = 30° and ∠EBC = 60°
If AD = xmand DE = hm, then CE = (h-16)m.

In the right ΔBAD,we have
`(AB)/(AD) = tan 30° = 1/ sqrt(3)`
`⇒16/x = 1/ sqrt(3)`
` ⇒x = 16 sqrt(3)=27.68m`
In the right ΔEBC,we have:
`(EC)/(BC) = tan 60^0 = sqrt(3)`
`⇒((h-16))/x = sqrt(3)`
`⇒ h - 16 = xsqrt(3)`
`⇒ h - 16=16 sqrt(3) xx sqrt(3) = 48 [ ∵ x = 16 sqrt(3)]`
`⇒ h = 48+16 = 64 m`
∴ Distance of the cliff from the deck of the ship = AD = x = 27.68m
And,
Height of the cliff = DE = h =64m
APPEARS IN
संबंधित प्रश्न
A 1.5 m tall boy is standing at some distance from a 30 m tall building. The angle of elevation from his eyes to the top of the building increases from 30° to 60° as he walks towards the building. Find the distance he walked towards the building.
A straight highway leads to the foot of a tower. A man standing at the top of the tower observes a car as an angle of depression of 30°, which is approaching the foot of the tower with a uniform speed. Six seconds later, the angle of depression of the car is found to be 60°. Find the time taken by the car to reach the foot of the tower from this point.
A tree breaks due to the storm and the broken part bends so that the top of the tree touches the ground making an angle of 30° with the ground. The distance from the foot of the tree to the point where the top touches the ground is 10 metres. Find the height of the tree.
Find the angle of elevation of the sum (sun's altitude) when the length of the shadow of a vertical pole is equal to its height.
If the height of a vertical pole is 3–√3 times the length of its shadow on the ground, then the angle of elevation of the Sun at that time is
(A) 30°
(B) 60°
(C) 45°
(D) 75°
From the top of the tower 60 m high the angles of depression of the top and bottom of a vertical lamp post are observed to be 38° and 60° respectively. Find the height of the lamp post (tan 38° = 0.7813, `sqrt(3)` = 1.732)
Three villagers A, B and C can see each other using telescope across a valley. The horizontal distance between A and B is 8 km and the horizontal distance between B and C is 12 km. The angle of depression of B from A is 20° and the angle of elevation of C from B is 30°. Calculate the vertical height between A and B. (tan 20° = 0.3640, `sqrt3` = 1.732)

An aeroplane is flying parallel to the Earth’s surface at a speed of 175 m/sec and at a height of 600 m. The angle of elevation of the aeroplane from a point on the Earth’s surface is 37°. After what period of time does the angle of elevation increase to 53°? (tan 53° = 1.3270, tan 37° = 0.7536)
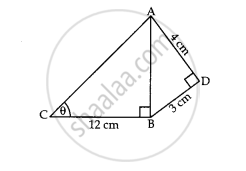
In given figure, AD = 4 cm, BD = 3 cm and CB = 12 cm. The value of tan `theta` is ____________.
An aeroplane at an altitude of 200 metres observes the angles of depression of opposite points on the two banks of a river to be 45° and 60°. Find the width of the river. (Use = `sqrt(3)` = 1.732)
