Advertisements
Advertisements
प्रश्न
A man goes 18 m due east and then 24 m due north. Find the distance of his current position from the starting point?
उत्तर
Let the initial position of the man be “O” and his final position be “B”.
By Pythagoras theorem
In the right ∆OAB,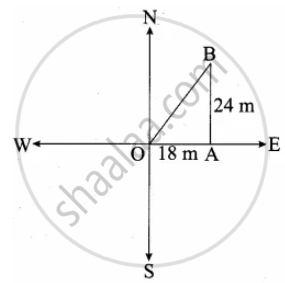
OB2 = OA2 + AB2
= 182 + 242
= 324 + 576 = 900
OB = `sqrt(900)` = 30
The distance of his current position is 30 m
APPEARS IN
संबंधित प्रश्न
Sides of triangle are given below. Determine it is a right triangle or not? In case of a right triangle, write the length of its hypotenuse. 7 cm, 24 cm, 25 cm
Identify, with reason, if the following is a Pythagorean triplet.
(3, 5, 4)
If the angles of a triangle are 30°, 60°, and 90°, then shown that the side opposite to 30° is half of the hypotenuse, and the side opposite to 60° is `sqrt(3)/2` times of the hypotenuse.
From the given figure, find the length of hypotenuse AC and the perimeter of ∆ABC.
AD is perpendicular to the side BC of an equilateral ΔABC. Prove that 4AD2 = 3AB2.
Two trains leave a railway station at the same time. The first train travels due west and the second train due north. The first train travels at a speed of `(20 "km")/"hr"` and the second train travels at `(30 "km")/"hr"`. After 2 hours, what is the distance between them?
Rithika buys an LED TV which has a 25 inches screen. If its height is 7 inches, how wide is the screen? Her TV cabinet is 20 inches wide. Will the TV fit into the cabinet? Give reason
In the given figure, AD is a median of a triangle ABC and AM ⊥ BC. Prove that:

(i) `"AC"^2 = "AD"^2 + "BC"."DM" + (("BC")/2)^2`
(ii) `"AB"^2 = "AD"^2 - "BC"."DM" + (("BC")/2)^2`
(iii) `"AC"^2 + "AB"^2 = 2"AD"^2 + 1/2"BC"^2`
Two angles are said to be ______, if they have equal measures.
Height of a pole is 8 m. Find the length of rope tied with its top from a point on the ground at a distance of 6 m from its bottom.
