Advertisements
Advertisements
प्रश्न
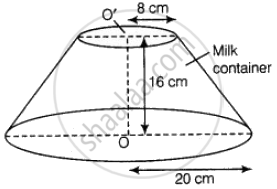
A milk container of height 16 cm is made of metal sheet in the form of frustum of a cone with radii of its lower and upper ends as 8 cm and 20 cm respectively. Find the cost of milk at the rate of ₹ 22 per litre which the container can hold.
उत्तर
Given that, height of milk container (h) = 16 cm,
Radius of lower end of milk container (r) = 8 cm
And radius of upper end of milk container (R) = 20 cm
∴ Volume of the milk container made of metal sheet in the form of a frustum of a cone
= `(πh)/3 (R^2 + r^2 + Rr)`
= `22/7 xx 16/3 [(20)^2 + (8)^2 + 20 xx 8]`
= `(22 xx 16)/21 (400 + 64 + 160)`
= `(22 xx 16 xx 624)/21`
= `219648/21`
= 10459.42 cm3 ......[∵ 1L = 1000 cm3]
= 10.45942 L
So, volume of the milk container is 10459.42 cm3
∵ Cost of 1 L milk = ₹ 22
∴ Cost of 10.45942 L milk = 22 × 10.45942 = ₹ 230.12
Hence, the required cost of milk is ₹ 230.12
APPEARS IN
संबंधित प्रश्न
A 5 m wide cloth is used to make a conical tent of base diameter 14 m and height 24 m. Find the cost of cloth used at the rate of Rs 25 per metre ?\[[Use \pi = \frac{22}{7}]\]
A metallic bucket, open at the top, of height 24 cm is in the form of the frustum of a cone, the radii of whose lower and upper circular ends are 7 cm and 14 cm respectively. Find :
(i) the volume of water which can completely fill the bucket.
(ii) the area of the metal sheet used to make the bucket.
[Use π =\[\frac{22}{7}\]
A solid toy s in the form of a hemisphere surrounded by a right circular cone . The height of cone is 4 cm and the diameter of the base is 8 cm . Determine the volume of the toy. If a cube circumscribes the toy , then find the difference of the volumes of cube and the toy .
A frustum of a cone is 9 cm thick and the diameters of its circular ends are 28 cm and 4 cm. Find the volume and lateral surface area of the frustum.
(Take π = 22/7).
If a cone and a sphere have equal radii and equal volumes. What is the ratio of the diameter of the sphere to the height of the cone?
If the areas of circular bases of a frustum of a cone are 4 cm2 and 9 cm2 respectively and the height of the frustum is 12 cm. What is the volume of the frustum?
A metallic sphere of radius 10.5 cm is melted and then recast into small cones, each of radius 3.5 cm and height 3 cm. The number of such cones is
The material of a cone is converted into the shape of a cylinder of equal radius. If height of the cylinder is 5 cm, then height of the cone is
A reservoir is in the shape of a frustum of a right circular cone. It is 8 m across at the top and 4 m across at the bottom. If it is 6 m deep, then its capacity is
A hollow sphere of internal and external diameters 4 cm and 8 cm respectively is melted into a cone of base diameter 8 cm. The height of the cone is
The maximum volume of a cone that can be carved out of a solid hemisphere of radius r is
The radii of the circular ends of a frustum are 6 cm and 14 cm. If its slant height is 10 cm, then its vertical height is
In a right circular cone , the cross-section made by a plane parallel to the base is a
The perimeters of the two circular ends of a frustum of a cone are 48 cm and 36 cm. If the height of the frustum is 11 cm, then find its volume and curved surface area.
The shape of a glass (tumbler) is usually in the form of

The base radii of two circular cones of the same height are in the ratio 3 : 5. The ratio of their volumes are ______.
The radii of the top and bottom of a bucket of slant height 13 cm are 9 cm and 4 cm respectively. The height of the bucket is ______.
A bucket is in the form of a frustum of a cone and holds 28.490 litres of water. The radii of the top and bottom are 28 cm and 21 cm, respectively. Find the height of the bucket.
Find the total surface area of frustum, if its radii are 15 cm and 7 cm. Also, the slant height of the frustum is 14 cm.
Radii of the frustum = `square` cm and `square` cm
Slant height of the frustum = `square` cm
Total surface area = `π[(r_1^2 + r_2^2 + (r_1 + r_2)l]`
= `22/7 [square + square + (square + square) square]`
= `22/7 (square)`
= `square` cm2
Hence, the total surface area of the frustum is `square`.
