Question

A parallel beam of monochromatic light is used in a Young's double slit experiment. The slits are separated by a distance d and the screen is placed parallel to the plane of the slits. Slow that if the incident beam makes an angle \[\theta =  \sin^{- 1}   \left( \frac{\lambda}{2d} \right)\] with the normal to the plane of the slits, there will be a dark fringe at the centre P₀ of the pattern.

Answer 1

Let the two slits are S₁ and S₂ with separation d as shown in figure.

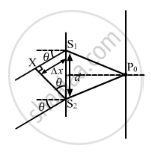
The wave fronts reaching P₀ from S₁ and S₂ will have a path difference of S₁X = ∆x.

In ∆S₁S₂X,

\[\sin\theta = \frac{S_1 X}{S_1 S_2} = \frac{∆ x}{d}\]

\[\Rightarrow  ∆ x = d\sin\theta\]

Using \[\theta =  \sin^{- 1}   \left( \frac{\lambda}{2d} \right)\] we get,

\[\Rightarrow  ∆ x = d \times \frac{\lambda}{2d} = \frac{\lambda}{2}\]

Hence, there will be dark fringe at point P₀ as the path difference is an odd multiple of \[\frac{\lambda}{2}.\]